We prove continuity in generalized parabolic Morrey spaces 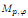 of sublinear operators generated by the parabolic Calderón—Zygmund operator and by the commutator of this operator with bounded mean oscillation (BMO) functions. As a consequence, we obtain a global
of sublinear operators generated by the parabolic Calderón—Zygmund operator and by the commutator of this operator with bounded mean oscillation (BMO) functions. As a consequence, we obtain a global 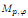 -regularity result for the Cauchy—Dirichlet problem for linear uniformly parabolic equations with vanishing mean oscillation (VMO) coefficients.
-regularity result for the Cauchy—Dirichlet problem for linear uniformly parabolic equations with vanishing mean oscillation (VMO) coefficients.