Associated with any closed quantum subgroup  $G\,\subset \,U_{N}^{+}$ and any index set
$G\,\subset \,U_{N}^{+}$ and any index set 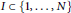 $I\,\subset \,\{1,\,.\,.\,.\,,\,N\}$ is a certain homogeneous space
$I\,\subset \,\{1,\,.\,.\,.\,,\,N\}$ is a certain homogeneous space 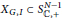 ${{X}_{G,I}}\subset S_{\mathbb{C},+}^{N-1},$ called an affine homogeneous space. Using Tannakian duality methods, we discuss the abstract axiomatization of the algebraic manifolds
${{X}_{G,I}}\subset S_{\mathbb{C},+}^{N-1},$ called an affine homogeneous space. Using Tannakian duality methods, we discuss the abstract axiomatization of the algebraic manifolds 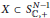 $X\subset S_{\mathbb{C},+}^{N-1}$ that can appear in this way.
$X\subset S_{\mathbb{C},+}^{N-1}$ that can appear in this way.