We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let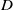 
$D$ be a division algebra, finite-dimensional over its center, and 
$R=D[t;\;\sigma,\delta ]$ a skew polynomial ring.
Using skew polynomials 
$f\in R$, we construct division algebras and maximum rank distance codes consisting of matrices with entries in a noncommutative division algebra or field. These include Jha Johnson semifields, and the classes of classical and twisted Gabidulin codes constructed by Sheekey.
