In this paper we establish that Hankel multipliers of Laplace transform type are bounded from
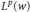 ${{L}^{p}}\left( w \right)$
into itself when
${{L}^{p}}\left( w \right)$
into itself when 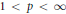 $1\,<\,p\,<\infty$, and from
$1\,<\,p\,<\infty$, and from
 ${{L}^{1}}\left( w \right)$
into
${{L}^{1}}\left( w \right)$
into
 ${{L}^{1,\infty }}\left( w \right)$
, provided that
${{L}^{1,\infty }}\left( w \right)$
, provided that  $w$ is in the Muckenhoupt class
$w$ is in the Muckenhoupt class
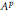 ${{A}^{p}}$ on
${{A}^{p}}$ on 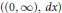 $\left( \left( 0,\,\infty \right),\,dx \right)$.
$\left( \left( 0,\,\infty \right),\,dx \right)$.
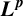 $\boldsymbol {L}^{\boldsymbol {p}}$–
$\boldsymbol {L}^{\boldsymbol {p}}$–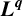 $\boldsymbol {L}^{\boldsymbol {q}}$ MULTIPLIERS ON COMMUTATIVE HYPERGROUPS
$\boldsymbol {L}^{\boldsymbol {q}}$ MULTIPLIERS ON COMMUTATIVE HYPERGROUPS