In this paper we use Langlands-Shahidi method and the result of Langlands which says that non self-conjugate maximal parabolic subgroups do not contribute to the residual spectrum, to prove the holomorphy of several completed automorphic  $L$-functions on the whole complex plane which appear in constant terms of the Eisenstein series. They include the exterior square
$L$-functions on the whole complex plane which appear in constant terms of the Eisenstein series. They include the exterior square  $L$-functions of
$L$-functions of 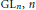 $\text{G}{{\text{L}}_{\text{n}}},\,n$ odd, the Rankin-Selberg
$\text{G}{{\text{L}}_{\text{n}}},\,n$ odd, the Rankin-Selberg  $L$-functions of
$L$-functions of 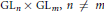 $\text{G}{{\text{L}}_{n}}\times \,\text{G}{{\text{L}}_{m}},\,n\,\ne \,m$, and
$\text{G}{{\text{L}}_{n}}\times \,\text{G}{{\text{L}}_{m}},\,n\,\ne \,m$, and  $L$-functions
$L$-functions 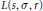 $L\left( s,\,\sigma ,\,r \right)$, where
$L\left( s,\,\sigma ,\,r \right)$, where  $\sigma $ is a generic cuspidal representation of
$\sigma $ is a generic cuspidal representation of 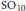 $\text{S}{{\text{O}}_{10}}$ and
$\text{S}{{\text{O}}_{10}}$ and  $r$ is the half-spin representation of GSpin
$r$ is the half-spin representation of GSpin 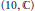 $\left( 10,\,\mathbb{C} \right)$. The main part is proving the holomorphy and non-vanishing of the local normalized intertwining operators by reducing them to natural conjectures in harmonic analysis, such as standard module conjecture.
$\left( 10,\,\mathbb{C} \right)$. The main part is proving the holomorphy and non-vanishing of the local normalized intertwining operators by reducing them to natural conjectures in harmonic analysis, such as standard module conjecture.