Hostname: page-component-669899f699-g7b4s Total loading time: 0 Render date: 2025-05-03T07:01:48.790Z Has data issue: false hasContentIssue false
  • English
  • Français

Normalized ground state solutions for critical growth Schrödinger equations with Hardy potential

Published online by Cambridge University Press:  09 December 2024

Song Fan ,
Gui-Dong Li  and
Chun-Lei Tang
Song Fan
Affiliation:
School of Mathematics and Statistics, Guizhou University, Guiyang 550025, People’s Republic of China ([email protected])
Gui-Dong Li
Affiliation:
School of Mathematics and Statistics, Guizhou University, Guiyang 550025, People’s Republic of China ([email protected])
Chun-Lei Tang*
Affiliation:
School of Mathematics and Statistics, Southwest University, Chongqing 400715, People’s Republic of China ([email protected]) (corresponding author)
*
*Corresponding author.
Get access Rights & Permissions [Opens in a new window]

Abstract

In this article, we study the following Schrödinger equation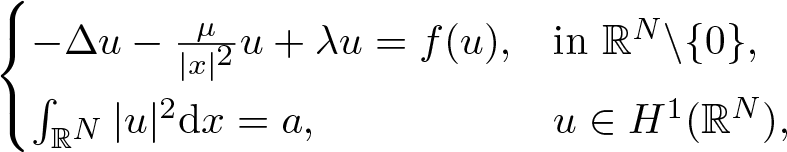

\begin{align*}\begin{cases}-\Delta u -\frac{\mu}{|x|^2} u+\lambda u =f(u), &\text{in}~ \mathbb{R}^N\backslash\{0\},\\\int_{\mathbb{R}^{N}}|u|^{2}\mathrm{d} x=a, & u\in H^1(\mathbb{R}^{N}),\end{cases}\end{align*}

where $N\geq 3$, a > 0, and 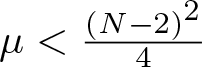$\mu \lt \frac{(N-2)^2}{4}$. Here 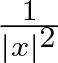$\frac{1}{|x|^2} $ represents the Hardy potential (or ‘inverse-square potential’), λ is a Lagrange multiplier, and the nonlinearity function f satisfies the general Sobolev critical growth condition. Our main goal is to demonstrate the existence of normalized ground state solutions for this equation when 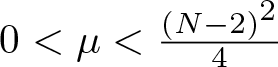$0 \lt \mu \lt \frac{(N-2)^2}{4}$. We also analyse the behaviour of solutions as $\mu\to0^+$ and derive the existence of normalized ground state solutions for the limiting case where µ = 0. Finally, we investigate the existence of normalized solutions when µ < 0 and analyse the asymptotic behaviour of solutions as $\mu\to 0^-$.

Keywords

asymptotic behaviourHardy potentialSobolev critical growthvariational methodsnormalized solutions
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , First View , pp. 1 - 30
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

*

This work was supported by Natural Science Research Project of Guizhou Province(No. QKHJC-ZK[2023]YB033, [2023]YB036) and National Natural Science Foundation of China (No.12201147, No.12371120)

References

Akhmediev, N. and Ankiewicz, A.. Partially coherent solitons on a finite background. Phys. Rev. Lett. 82 (1999), 2661–2664.CrossRefGoogle Scholar
Alves, C. O., Ji, C. and Miyagaki, O. H.. Normalized solutions for a Schrödinger equation with critical growth in $\mathbb{R}^N$. Calc. Var. Partial Differential Equations 61 (2022), .CrossRefGoogle Scholar
Bahouri, H., Chemin, J.-Y. and Danchin, R.. Fourier analysis and nonlinear partial differential equations, Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences], (Springer, Heidelberg, 2011).Google Scholar
Bartsch, T. and de Valeriola, S.. Normalized solutions of nonlinear Schrödinger equations. Arch. Math. (Basel) 100 (2013), 75–83.CrossRefGoogle Scholar
Bartsch, T., Molle, R., Rizzi, M. and Verzini, G.. Normalized solutions of mass supercritical Schrödinger equations with potential. Comm. Partial Differential Equations 46 (2021), 1729–1756.CrossRefGoogle Scholar
Bartsch, T. and Soave, N.. A natural constraint approach to normalized solutions of nonlinear Schrödinger equations and systems. J. Funct. Anal. 272 (2017), 4998–5037.CrossRefGoogle Scholar
Bartsch, T. and Soave, N.. Multiple normalized solutions for a competing system of Schrödinger equations. Calc. Var. Partial Differential Equations 58 (2019), .CrossRefGoogle Scholar
Berestycki, H. and Cazenave, T.. Instabilité des états stationnaires dans les équations de Schrödinger et de Klein-Gordon non linéaires. C. R. Acad. Sci. Paris Sér. I Math. 293 (1981), 489–492.Google Scholar
Berestycki, H. and Esteban, M. J.. Existence and bifurcation of solutions for an elliptic degenerate problem. J. Differential Equations 134 (1997), 1–25.CrossRefGoogle Scholar
Berestycki, H. and Lions, P.-L.. Nonlinear scalar field equations. I. Existence of a ground state. Arch. Rational Mech. Anal. 82 (1983), 313–345.CrossRefGoogle Scholar
Bieganowski, B. and Mederski, J.. Normalized ground states of the nonlinear Schrödinger equation with at least mass critical growth. J. Funct. Anal. 280 (2021), .CrossRefGoogle Scholar
Bieganowski, B., Mederski, J. and Schino, J.. Normalized solutions to at least mass critical problems: singular polyharmonic equations and related curl-curl problems. J. Geom. Anal. 34 (2024), .CrossRefGoogle Scholar
Brézis, H. and Lieb, E.. A relation between pointwise convergence of functions and convergence of functionals. Pmc. Amer. Math. Soc. 88 (1983), 486–490.CrossRefGoogle Scholar
Buryak, A. V., Di Trapani, P., Skryabin, D. V. and Trillo, S.. Optical solitons due to quadratic nonlinearities: from basic physics to futuristic applications. Phys. Rep. 370 (2002), 63–235.CrossRefGoogle Scholar
Cazenave, T. and Lions, P.-L.. Orbital stability of standing waves for some nonlinear Schrödinger equations. Comm. Math. Phys. 85 (1982), 549–561.CrossRefGoogle Scholar
Chen, J., Li, S. and Li, Y.. Multiple solutions for a semilinear equation involving singular potential and critical exponent. Z. Angew. Math. Phys. 56 (2005), 453–474.CrossRefGoogle Scholar
Chou, K. S. and Chu, C. W.. On the best constant for a weighted Sobolev-Hardy inequality. J. London Math. Soc. (2) 48 (1993), 137–151.CrossRefGoogle Scholar
Ding, Y. and Zhong, X.. Normalized solution to the Schrödinger equation with potential and general nonlinear term: mass super-critical case. J. Differential Equations 334 (2022), 194–215.CrossRefGoogle Scholar
Ferrero, A. and Gazzola, F.. Existence of solutions for singular critical growth semilinear elliptic equations. J. Differential Equations 177 (2001), 494–522.CrossRefGoogle Scholar
Frantzeskakis, D. J.. Dark solitons in atomic Bose-Einstein condensates: from theory to experiments. J. Phys. A 43 (2010), .CrossRefGoogle Scholar
Ghoussoub, N.. Duality and perturbation methods in critical point theory. Of Cambridge Tracts in Mathematics, Vol. 107 (With appendices by David Robinson, Cambridge, 1993)Google Scholar
Ghoussoub, N. and Robert, F.. The Hardy-Schrödinger operator with interior singularity: the remaining cases. Calc. Var. Partial Differ. Equ. 56 (2017), .CrossRefGoogle Scholar
Guo, Q. and Mederski, J.. Ground states of nonlinear Schrödinger equations with sum of periodic and inverse-square potentials. J. Differential Equations 260 (2016), 4180–4202.CrossRefGoogle Scholar
Han, P.. Asymptotic behavior of solutions to semilinear elliptic equations with Hardy potential. Proc. Amer. Math. Soc. 135 (2007), 365–372.CrossRefGoogle Scholar
Hirata, J. and Tanaka, K.. Nonlinear scalar field equations with L 2 constraint: mountain pass and symmetric mountain pass approaches. Adv. Nonlinear Stud. 19 (2019), 263–290.CrossRefGoogle Scholar
Ikoma, N. and Miyamoto, Y.. Stable standing waves of nonlinear Schrödinger equations with potentials and general nonlinearities. Calc. Var. Partial Differential Equations 59 (2020), .CrossRefGoogle Scholar
Jeanjean, L.. Existence of solutions with prescribed norm for semilinear elliptic equations, Nonlinear Anal. 28 (1997), 1633–1659.CrossRefGoogle Scholar
Jeanjean, L., Jendrej, J., Le, T. T. and Visciglia, N.. Orbital stability of ground states for a Sobolev critical Schrödinger equation. J. Math. Pures Appl. (9) 164 (2022), 158–179.CrossRefGoogle Scholar
Jeanjean, L. and Le, T. T.. Multiple normalized solutions for a Sobolev critical Schrödinger equation. Math. Ann. 384 (2022), 101–134.CrossRefGoogle Scholar
Jeanjean, L. and Lu, S.-S.. Nonradial normalized solutions for nonlinear scalar field equations. Nonlinearity 32 (2019), 4942–4966.CrossRefGoogle Scholar
Jeanjean, L. and Lu, S.-S.. A mass supercritical problem revisited. Calc. Var. Partial Differential Equations 59 (2020), .CrossRefGoogle Scholar
Jeanjean, L., Zhang, J. and Zhong, X.. A global branch approach to normalized solutions for the Schrödinger equation. J. Math. Pures Appl. (9) 183 (2024), 44–75.CrossRefGoogle Scholar
Jia, H. and Luo, X.. Prescribed mass standing waves for energy critical Hartree equations. Calc. Var. Partial Differential Equations 62 (2023), .CrossRefGoogle Scholar
Li, G.-D., Li, Y.-Y. and Tang, C.-L.. Ground state solutions for critical Schrödinger equations with Hardy potential. Nonlinearity 35 (2022), 5076–5108.CrossRefGoogle Scholar
Li, H. and Zou, W.. Normalized ground state for the Sobolev critical Schrödinger equation involving Hardy term with combined nonlinearities. Math. Nachr. 296 (2023), 2440–2466.CrossRefGoogle Scholar
Li, X.. Existence of normalized ground states for the Sobolev critical Schrödinger equation with combined nonlinearities. Calc. Var. Partial Differential Equations 60 (2021), .CrossRefGoogle Scholar
Lions, P.-L.. The concentration-compactness principle in the calculus of variations. The locally compact case. II. Ann Inst. H. Poincaré Anal. Non Linéaire 1 (1984), 223–283.CrossRefGoogle Scholar
Liu, M. and Chang, X.. Normalized ground state solutions for nonlinear Schrödinger equations with general Sobolev critical nonlinearities. Discrete Contin. Dyn. Syst. Ser. S., 10.3934/dcdss.2024035OI.Google Scholar
Molle, R., Riey, G. and Verzini, G.. Normalized solutions to mass supercritical Schrödinger equations with negative potential. J. Differential Equations 333 (2022), 302–331.CrossRefGoogle Scholar
Pellacci, B., Pistoia, A., Vaira, G. and Verzini, G.. Normalized concentrating solutions to nonlinear elliptic problems. J. Differential Equations 275 (2021), 882–919.CrossRefGoogle Scholar
Reed, M. and Simon, B.. Methods of Modern Mathematical Physics. I. 2nd ed. (Academic Press, Inc. [Harcourt Brace Jovanovich, Publishers], New York, 1980) Functional analysis.Google Scholar
Shibata, M.. Stable standing waves of nonlinear Schrödinger equations with a general nonlinear term. Manuscripta Math. 143 (2014), 221–237.CrossRefGoogle Scholar
Soave, N.. Normalized ground states for the NLS equation with combined nonlinearities. J. Differential Equations 269 (2020), 6941–6987.CrossRefGoogle Scholar
Soave, N.. Normalized ground states for the NLS equation with combined nonlinearities: the Sobolev critical case. J. Funct. Anal. 279 (2020), .CrossRefGoogle Scholar
Szulkin, A. and Weth, T.. Ground state solutions for some indefinite variational problems. J. Funct. Anal. 257 (2009), 3802–3822.CrossRefGoogle Scholar
Szulkin, A. and Weth, T.. The Method of Nehari Manifold, in Handbook of Nonconvex Analysis and Applications, 597–632 (Int. Press, Somerville, MA, 2010).Google Scholar
Talenti, G.. Best constant in Sobolev inequality. Ann. Mat. Pura Appl. (4) 110 (1976), 353–372.CrossRefGoogle Scholar
Timmermans, E.. Phase separation of Bose-Einstein condensates. Phys. Rev. Lett. 81 (1998), 5718–5721.CrossRefGoogle Scholar
Wei, J. and Wu, Y.. Normalized solutions for Schrödinger equations with critical Sobolev exponent and mixed nonlinearities. J. Funct. Anal. 283 (2022), .CrossRefGoogle Scholar
Willem, M.. Minimax theorems. Of Progress in Nonlinear Differential Equations and Their Applications, Vol. 24 (Birkhäuser Boston, Inc., Boston, MA, 1996)Google Scholar
Yang, Z.. A new observation for the normalized solution of the Schrödinger equation. Arch. Math. (Basel) 115 (2020), 329–338.CrossRefGoogle Scholar
Zhou, L., Zhang, C., Zhang, L. and Zhou, L., Normalized multibump solutions to nonlinear Schrödinger equations with steep potential well. Nonlinearity 35 (2022), 4624–4658.Google Scholar