Hostname: page-component-669899f699-2mbcq Total loading time: 0 Render date: 2025-04-27T22:32:48.409Z Has data issue: false hasContentIssue false
  • English
  • Français

Effect of the location of a protection zone in a reaction–diffusion model

Part of: Parabolic equations and systems Partial differential equations

Published online by Cambridge University Press:  14 June 2023

Ningkui Sun
Ningkui Sun*
Affiliation:
School of Mathematics and Statistics, Shandong Normal University, Jinan 250014, China ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we consider the dynamical behaviour of a reaction–diffusion model for a population residing in a one-dimensional habit, with emphasis on the effects of boundary conditions and protection zone. We assume that the population is subjected to a strong Allee effect in its natural domain but obeys a monostable nonlinear growth in the protection zone $[L_1,\, L_2]$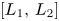 with two constants satisfying $0\leq L_1< L_2$, and the general Robin condition is imposed on $x=0$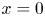 (i.e. $u(t,\,0)=bu_x(t,\,0)$ with $b\geq 0$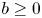). We show the existence of two critical values $0< L_*\leq L^*$, and prove that a vanishing–transition–spreading trichotomy result holds when the length of protection zone is smaller than $L_*$; a transition–spreading dichotomy result holds when the length of protection zone is between $L_*$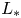 and $L^*$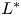; only spreading happens when the length of protection zone is larger than $L^*$. Based on the properties of $L_*$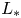, we obtain the precise strategies for an optimal protection zone: if $b$ is large (i.e. $b\geq 1/\sqrt {-g'(0)}$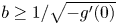), the protection zone should start from somewhere near $0$; while if $b$ is small (i.e. 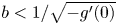$b< 1/\sqrt {-g'(0)}$), then the protection zone should start from somewhere away from $0$, and as far away from $0$ as possible.

Keywords

Reaction–diffusion equationstrong Allee effectprotection zonespecies spreadinglong-time behaviour

MSC classification

Primary: 35K15: Initial value problems for second-order parabolic equations
Secondary: 35K55: Nonlinear parabolic equations 35B40: Asymptotic behavior of solutions 92D25: Population dynamics (general)
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 154 , Issue 4 , August 2024 , pp. 1095 - 1117
Check for updates
Copyright
Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Allee, W. C.. Animal aggregations: a study in general sociology (Chicago: University of Chicago Press, 1931).10.5962/bhl.title.7313CrossRefGoogle Scholar
Aronson, D. G. and Weinberger, H. F.. Multidimensional nonlinear diffusion arising in population genetics. Adv. Math. 30 (1978), 33–76.10.1016/0001-8708(78)90130-5CrossRefGoogle Scholar
Berestycki, H., Hamel, F. and Rossi, L.. Liouville-type results for semilinear elliptic equations in unbounded domains. Ann. Mat. Pura Appl. 186 (2007), 469–507.10.1007/s10231-006-0015-0CrossRefGoogle Scholar
Berestycki, H. and Rossi, L.. On the principal eigenvalue of elliptic operators in $\mathbb {R}^N$ and applications. J. Eur. Math. Soc. 8 (2006), 195–215.10.4171/jems/47CrossRefGoogle Scholar
Chen, X., Lou, B., Zhou, M. and Giletti, T.. Long time behavior of solutions of a reaction–diffusion equation on bounded intervals with Robin boundary conditions. Ann. I. H. Poincaré-AN 33 (2016), 67–92.10.1016/j.anihpc.2014.08.004CrossRefGoogle Scholar
Courchamp, F., Berec, L. and Gascoigne, J.. Allee effects in ecology and conservation (Oxford, New York, USA: Oxford University Press, 2008).10.1093/acprof:oso/9780198570301.001.0001CrossRefGoogle Scholar
Cui, R., Li, H., Mei, L. and Shi, J.-P.. Effect of harvesting quota and protection zone in a reaction–diffusion model arising from fishery management. Discrete Contin. Dyn. Syst. Ser. B 22 (2017), 791–807.Google Scholar
Cui, R., Shi, J.-P. and Wu, B.. Strong Allee effect in a diffusive predator–prey system with a protection zone. J. Differ. Equ. 256 (2014), 108–129.10.1016/j.jde.2013.08.015CrossRefGoogle Scholar
Dieu, N. T., Du, N. H., Nguyen, H. D. and Yin, G.. Protection zones for survival of species in random environment. SIAM J. Appl. Math. 76 (2016), 1382–1402.10.1137/15M1032004CrossRefGoogle Scholar
Du, K., Peng, R. and Sun, N.. The role of protection zone on species spreading governed by a reaction–diffusion model with strong Allee effect. J. Differ. Equ. 266 (2019), 7327–7356.10.1016/j.jde.2018.11.035CrossRefGoogle Scholar
Du, Y. and Liang, X.. A diffusive competition model with a protection zone. J. Differ. Equ. 244 (2008), 61–86.10.1016/j.jde.2007.10.005CrossRefGoogle Scholar
Du, Y., Lou, B., Peng, R. and Zhou, M.. The Fisher–KPP equation over simple graphs: varied persistence states in river networks. J. Math. Biol. 80 (2020), 1559–1616.10.1007/s00285-020-01474-1CrossRefGoogle ScholarPubMed
Du, Y., Peng, R. and Wang, M.. Effect of a protection zone in the diffusive Leslie predator–prey model. J. Differ. Equ. 246 (2009), 3932–3956.10.1016/j.jde.2008.11.007CrossRefGoogle Scholar
Du, Y. and Matano, H.. Convergence and sharp thresholds for propagation in nonlinear diffusion problems. J. Eur. Math. Soc. 12 (2010), 279–312.10.4171/jems/198CrossRefGoogle Scholar
Du, Y. and Shi, J.-P.. A diffusive predator–prey model with a protection zone. J. Differ. Equ. 229 (2006), 63–91.10.1016/j.jde.2006.01.013CrossRefGoogle Scholar
Du, Y. and Shi, J.-P., Some recent results on diffusive predator–prey models in spatially heterogeneous environment. Nonlinear Dynamics and Evolution Equations, Fields Inst. Commun., Vol. 48 (Amer. Math. Soc., Providence, RI, 2006), pp. 95–135.10.1090/fic/048/05CrossRefGoogle Scholar
He, X. and Zheng, S. N.. Protection zone in a diffusive predator–prey model with Beddington–DeAngelis functional response. J. Math. Biol. 75 (2017), 239–257.10.1007/s00285-016-1082-5CrossRefGoogle Scholar
He, X. and Zheng, S. N.. Protection zone in a modified Lotka–Volterra model. Discrete Contin. Dyn. Syst. Ser. B 20 (2015), 2027–2038.Google Scholar
Jin, Y., Peng, R. and Shi, J.-P.. Population dynamics in river networks. J. Nonlinear Sci. 29 (2019), 2501–2545.10.1007/s00332-019-09551-6CrossRefGoogle Scholar
Keitt, T. H., Lewis, M. A. and Holt, R. D.. Allee effects, invasion pinning, and species’ borders. Am. Naturalist 157 (2001), 203–216.10.1086/318633CrossRefGoogle ScholarPubMed
Lam, K. Y., Lou, Y. and Lutscher, F.. Evolution of dispersal in closed advective environments. J. Biol. Dyn. 9 (2015), 188–212.10.1080/17513758.2014.969336CrossRefGoogle ScholarPubMed
Lam, K. Y., Lou, Y. and Lutscher, F.. The emergence of range limits in advective environments. SIAM J. Appl. Math. 76 (2016), 641–662.10.1137/15M1027887CrossRefGoogle Scholar
Lewis, M. A. and Kareiva, P.. Allee dynamics and the spread of invading organisms. Theor. Popul. Biol. 43 (1993), 141–158.10.1006/tpbi.1993.1007CrossRefGoogle Scholar
Li, S. and Wu, J.. Effect of cross-diffusion in the diffusion prey–predator model with a protection zone. Discrete Contin. Dyn. Syst. 37 (2017), 1539–1558.10.3934/dcds.2017063CrossRefGoogle Scholar
Li, S., Wu, J. and Liu, S.. Effect of cross-diffusion on the stationary problem of a Leslie prey–predator model with a protection zone. Calc. Var. Partial Differ. Equ. 56 (2017), 35.10.1007/s00526-017-1159-zCrossRefGoogle Scholar
Li, S. and Yamada, Y.. Effect of cross-diffusion in the diffusion prey–predator model with a protection zone II. J. Math. Anal. Appl. 461 (2018), 971–992.10.1016/j.jmaa.2017.12.029CrossRefGoogle Scholar
Lou, Y. and Lutscher, F.. Evolution of dispersal in open advective environments. J. Math. Biol. 69 (2014), 1319–1342.10.1007/s00285-013-0730-2CrossRefGoogle ScholarPubMed
Maciel, G. A. and Lutscher, F.. Allee effects and population spread in patchy landscapes. J. Biol. Dyn. 9 (2015), 109–123.10.1080/17513758.2015.1027309CrossRefGoogle ScholarPubMed
Newman, M.. A model of mass extinction. J. Theor. Biol. 189 (1997), 235–252.10.1006/jtbi.1997.0508CrossRefGoogle Scholar
Oeda, K.. Effect of cross-diffusion on the stationary problem of a prey–predator model with a protection zone. J. Differ. Equ. 250 (2011), 3988–4009.10.1016/j.jde.2011.01.026CrossRefGoogle Scholar
Ramirez, J. M.. Population persistence under advection–diffusion in river networks. J. Math. Biol. 65 (2012), 919–942.10.1007/s00285-011-0485-6CrossRefGoogle ScholarPubMed
Sarhad, J., Manifold, S. and Anderson, K. E.. Geometric indicators of population persistence in branching continuous-space networks. J. Math. Biol. 74 (2017), 981–1009.10.1007/s00285-016-1045-xCrossRefGoogle ScholarPubMed
Sun, N.. A time-periodic reaction–diffusion–advection equation with a free boundary and sign-changing coefficients. Nonlinear Anal. Real World Appl. 51 (2020), 102952.10.1016/j.nonrwa.2019.06.002CrossRefGoogle Scholar
Sun, N. and Han, X.. Asymptotic behavior of solutions of a reaction–diffusion model with a protection zone and a free boundary. Appl. Math. Lett. 107 (2020), 106470.10.1016/j.aml.2020.106470CrossRefGoogle Scholar
Sun, N. and Lei, C.. Long-time behavior of a reaction–diffusion model with strong Allee effect and free boundary: effect of protection zone. J. Dynam. Differ. Equ. 35 (2023), 737–770.10.1007/s10884-021-10027-zCrossRefGoogle Scholar
Sun, N. and Li, J.. The effect of protection zone on asymptotic dynamics of a reaction–diffusion model with a free boundary or unbounded boundary. Nonlinear Anal. Real World Appl. 68 (2022), 103697.Google Scholar
Sun, N., Lou, B. and Zhou, M.. Fisher–KPP equation with free boundaries and time-periodic advections. Calc. Var. Partial Differ. Equ. 56 (2017), 36.10.1007/s00526-017-1165-1CrossRefGoogle Scholar
von Below, J.. Classical solvability of linear parabolic equations on networks. J. Differ. Equ. 72 (1988), 316–337.10.1016/0022-0396(88)90158-1CrossRefGoogle Scholar
Wang, J.-F., Shi, J.-P. and Wei, J.-J.. Dynamics and pattern formation in a diffusive predator–prey system with strong Allee effect in prey. J. Differ. Equ. 251 (2011), 1276–1304.10.1016/j.jde.2011.03.004CrossRefGoogle Scholar
Wang, Y. and Li, W.-T.. Effect of cross-diffusion on the stationary problem of a diffusive competition model with a protection zone. Nonlinear Anal. Real World Appl. 14 (2013), 224–245.10.1016/j.nonrwa.2012.06.001CrossRefGoogle Scholar
Wilson, E. O.. The future of life (Random House, 2002).Google Scholar
Zeng, X., Zeng, W. and Liu, L.. Effect of the protection zone on coexistence of the species for a ratio-dependent predator–prey model. J. Math. Anal. Appl. 462 (2018), 1605–1626.10.1016/j.jmaa.2018.02.060CrossRefGoogle Scholar
Zlatoš, A.. Sharp transition between extinction and propagation of reaction. J. Am. Math. Soc. 19 (2006), 251–263.10.1090/S0894-0347-05-00504-7CrossRefGoogle Scholar