Hostname: page-component-669899f699-2mbcq Total loading time: 0 Render date: 2025-04-30T04:27:52.393Z Has data issue: false hasContentIssue false
  • English
  • Français

On a quasilinear elliptic problem involving the 1-Laplacian operator and a discontinuous nonlinearity

Part of: Elliptic equations and systems

Published online by Cambridge University Press:  28 December 2022

Marcos T. O. Pimenta Open the ORCID record for Marcos T. O. Pimenta [Opens in a new window] ,
Gelson Conceição G. dos Santos  and
João R. Santos Júnior
Marcos T. O. Pimenta
Affiliation:
Departamento de Matemática e Computação, Universidade Estadual Paulista - Unesp, CEP: 19060-900, Presidente Prudente - SP, Brazil ([email protected])
Gelson Conceição G. dos Santos
Affiliation:
Faculdade de Matemática, Universidade Federal do Pará, CEP: 66075-110, Belém - PA, Brazil ([email protected], [email protected])
João R. Santos Júnior
Affiliation:
Faculdade de Matemática, Universidade Federal do Pará, CEP: 66075-110, Belém - PA, Brazil ([email protected], [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

In this work, we study a quasilinear elliptic problem involving the 1-Laplacian operator, with a discontinuous, superlinear and subcritical nonlinearity involving the Heaviside function $H(\cdot - \beta )$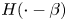. Our approach is based on an analysis of the associated p-Laplacian problem, followed by a thorough analysis of the asymptotic behaviour or such solutions as $p \to 1^+$. We study also the asymptotic behaviour of the solutions, as $\beta \to 0^+$ and we prove that it converges to a solution of the original problem, without the discontinuity in the nonlinearity.

Keywords

1-Laplacian operatorspace of functions of bounded variationdiscontinuous nonlinearities

MSC classification

Primary: 35J62: Quasilinear elliptic equations
Secondary: 35J75: Singular elliptic equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 154 , Issue 1 , February 2024 , pp. 33 - 59
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Alves, C. O., Yuan, Z. and Huang, L.. Existence and multiplicity of solutions for discontinuous elliptic problems in RN. Proc. R. Soc. Edinb. Sect. A Math. 151 (2020), 1–125.Google Scholar
Alves, C. O., dos Santos, J. A. and Nemer, R. C.. Multiple solutions for a problem with discontinuous nonlinearity. Ann. Mat. Pura Appl. 197 (2018), 883–903.CrossRefGoogle Scholar
Ambrosetti, A. and Badiale, M.. The dual variational principle and elliptic problems with discontinuous nonlinearities. J. Math. Anal. Appl. 140 (1989), 363–373.CrossRefGoogle Scholar
Ambrosetti, A., Calahorrano, M. and Dobarro, F.. Global branching for discontinuous problems. Comm.. Math. Univ. Carol. 31 (1990), 213–222.Google Scholar
Ambrosetti, A. and Turner, R. E. L.. Some discontinuous variational problems. Differ. Int. Equ. 1 (1988), 341–349.Google Scholar
Ambrosio, L., Fusco, N. and Pallara, D.. Functions of bounded variation and free discontinuity problems (Oxford: Oxford University Press, 2000).CrossRefGoogle Scholar
Andreu, F., Ballester, C., Caselles, V. and Mazón, J. M.. The Dirichlet problem for the total variation flow. J. Funct. Anal. 180 (2001), 347–403.CrossRefGoogle Scholar
Andreu, F., Ballester, C., Caselles, V. and Mazón, J. M.. Minimizing total variation flow. C. R. Acad. Sci., Paria, Sr. I, Math. 331 (2000), 867–872.CrossRefGoogle Scholar
Andreu, F., Ballester, C., Caselles, V. and Mazón, J. M.. Minimizing total variation flow. Differ. Int. Equ. 14 (2001), 321–360.Google Scholar
Andreu, F., Caselles, V. and Mazón, J. M., Parabolic Quasilinear Equations Minimizing Linear Growth Functionals, Progress in mathematics, Vol. 233 (Birkhäuser Verlag, Basel, 2004).Google Scholar
Anmin, M. and Chang, H.. Kirchhoff type problems in $\mathbb {R}^N$ with radial potentials and locally lipschitz functional. Appl. Math. Lett. 62 (2016), 49–54.Google Scholar
Anzellotti, G.. Pairings between measures and bounded functions and compensated compactness. Ann. Mat. Pura Appl. 4 (1983), 293–318.CrossRefGoogle Scholar
Arcoya, D. and Calahorrano, M.. Some discontinuos problems with a quasilinear operator. J. Math. Anal. Appl. 187 (1994), 1059–1072.CrossRefGoogle Scholar
Cerami, G.. Soluzioni positive di problemi con parte nonlineare discontinua e applicazioni a un problema di frontiera libera. Boll. UMI. 6 (1983), 321–338.Google Scholar
Chang, K. C.. The obstacle problem and partial differential equations with discontinuous nonlinearities. Commun Pure Appl Math. 33 (1978), 117–146.CrossRefGoogle Scholar
Chang, K. C.. On the multiple solutions of the elliptic differential equations with discontinuous nonlinear terms. Sci. Sin. 21 (1978), 139–158.Google Scholar
Chang, K. C.. Variational methods for nondifferentiable functionals and their applications to partial differential equations. J. Math. Anal. 80 (1981), 102–129.CrossRefGoogle Scholar
Chang, K.. The spectrum of the 1-Laplace operator. Commun. Contemp. Math. 9 (2009), 515–543.Google Scholar
Chen, G. and Frid, H.. Divergence-measure fields and hyperbolic conservation laws. Arch. Ration. Mech. Anal. 147 (1999), 89–118.CrossRefGoogle Scholar
Clarke, F. H.. Optimization and Nonsmooth Analysis (NY: John Wiley & Sons, 1983).Google Scholar
Clarke, F. H.. Generalized gradients and applications. Trans. Amer. Math. Soc. 265 (1975), 247–262.CrossRefGoogle Scholar
Demengel, F.. On some nonlinear partial differential equations involving the $1-$Laplacian and critical Sobolev exponent. ESAIM, Control Optim. Calc. Var. 4 (1999), 667–686.CrossRefGoogle Scholar
Figueiredo, G. M. and dos Santos, G. G.. Solution for a Kirchhoff equation with critical Caffarelli-Kohn-Nirenberg growth and discontinuous nonlinearity. Z. Angew. Math. Phys. 69 (2018), 7–5.Google Scholar
Gazzola, F. and Rădulescu, V.. A nonsmooth critical point theory approach to some nonlinear elliptic equations in $\mathbb {R}^N$. Differ. Int. Equ. 13 (2000), 47–60.Google Scholar
Gilbarg, D. and Trudinger, N. S.. I Elliptic Partial Differential Equations of Second-order (Berlin, Heidelberg, New York: Springer-Verlag, 1977).CrossRefGoogle Scholar
Grossinho, M. R. and Tersian, S. A.. An Introduction to Minimax Theorems and Their Applications to Differential Equations (Springer, Boston, MA, 2001).Google Scholar
Kawohl, B.. On a family of torsional creep problems. J. Reine Angew. Math. 410 (1990), 1–22.Google Scholar
Krawcewicz, W. and Marzantowicz, W.. Some remarks on the Lusternik- Schnirelman method for non differentiable functionals invariant with respect to a finite group action. Rocky Mountain J. Math. 20 (1990), 1041–1049.CrossRefGoogle Scholar
Mercaldo, A., Rossi, J. D., Segura de León, S. and Trombetti, C.. Behaviour of p-Laplacian problems with Neumann boundary conditions when p goes to 1. Commun. Pure Appl. Anal. 12 (2013), 253–267.CrossRefGoogle Scholar
Bisci, G. Molica and Repovš, D.. Some hemivariational inequalities in the Euclidean space. Adv. Nonlinear Anal. 9 (2020), 958–977.CrossRefGoogle Scholar
Moser, J.. A new proof de Giorgi's theorem concerning the regularity problem for elliptic differential equations. Comm. Pure Appl. Math. 13 (1960), 457–468.CrossRefGoogle Scholar
Segura de León, S. and Molino Salas, A.. Elliptic equations involving the $1-$Laplacian and a subcritical source term. Nonlinear Anal. 168 (2018), 50–66.Google Scholar
Szulkin, A.. Minimax principle for lower semicontinuous functions and applications to nonlinear boundary value problems. Ann. Inst. Henri Poincar 3 (1986), 77–109.CrossRefGoogle Scholar
Rădulescu, V.. Mountain pass theorems for non-differentiable functions and applications. Proc. Jpn. Acad., Ser. A, Math. Sci. 69 (1993), 193–198.CrossRefGoogle Scholar