Hostname: page-component-669899f699-swprf Total loading time: 0 Render date: 2025-04-29T06:55:24.807Z Has data issue: false hasContentIssue false
  • English
  • Français

Blowup of cylindrically symmetric solutions for biharmonic NLS

Part of: Partial differential equations Equations of mathematical physics and other areas of application

Published online by Cambridge University Press:  03 October 2024

Tianxiang Gou
Tianxiang Gou*
Affiliation:
School of Mathematics and Statistics, Xi’an Jiaotong University, Xi’an, Shaanxi, China
*
Email: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we consider blowup of solutions to the Cauchy problem for the following biharmonic nonlinear Schrödinger equation (NLS),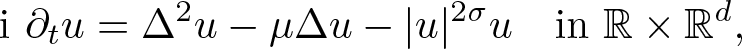

\begin{equation*}\text{i } \partial_t u=\Delta^2 u-\mu \Delta u-|u|^{2 \sigma} u \quad \text{in} \,\, \mathbb{R} \times \mathbb{R}^d,\end{equation*}

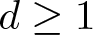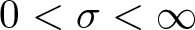where $d \geq 1$, $\mu \in \mathbb{R}$ and $0 \lt \sigma \lt \infty$ if 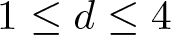$1 \leq d \leq 4$ and 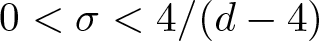$0 \lt \sigma \lt 4/(d-4)$ if 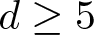$d \geq 5$. In the mass critical and supercritical cases, we establish the existence of blowup solutions to the problem for cylindrically symmetric data. The result extends the known ones with respect to blowup of solutions to the problem for radially symmetric data.

Keywords

blowupbiharmonic NLScylindrical symmetry solutions

MSC classification

Primary: 35Q55: NLS-like equations (nonlinear Schrödinger)
Secondary: 35B44: Blow-up 35B35: Stability
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 67 , Issue 4 , November 2024 , pp. 1085 - 1098
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Baruch, G. and Fibich, G., Singular solutions of the L 2-supercritical biharmonic nonlinear Schrödinger equation, Nonlinearity 24 (6) (2011), 1843–1859.CrossRefGoogle Scholar
Baruch, G., Fibich, G. and Mandelbaum, E., Ring-type singular solutions of the biharmonic nonlinear Schrödinger equation, Nonlinearity 23(11) (2010), 2867–2887.CrossRefGoogle Scholar
Baruch, G., Fibich, G. and Mandelbaum, E., Singular solutions of the biharmonic nonlinear Schrödinger equation, SIAM J. Appl. Math. 70(8) (2010), 3319–3341.CrossRefGoogle Scholar
Bellazzini, J. and Forcella, L., Dynamical collapse of cylindrical symmetric dipolar Bose-Einstein condensates, Calc. Var. Partial Differential Equations 60(229) (2021), .CrossRefGoogle Scholar
Bellazzini, J., Forcella, L. and Georgiev, V., Ground state energy threshold and blow-up for NLS with competing nonlinearities, Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 24(2) (2023), 955–988.Google Scholar
Bonheure, D., Castéras, J.-B., Gou, T. and Jeanjean, L., Normalized solutions to the mixed dispersion nonlinear Schrödinger equation in the mass critical and supercritical regime, Trans. Amer. Math. Soc. 372(3) (2019), 2167–2212.CrossRefGoogle Scholar
Bonheure, D., Castéras, J.-B., Gou, T. and Jeanjean, L., Strong instability of ground states to a fourth order Schrödinger equation, Int. Math. Res. Not. IMRN 2019(17) (2019), 5299–5315.CrossRefGoogle Scholar
Boulenger, T. and Lenzmann, E., Blowup for biharmonic NLS, Ann. Sci. Éc. Norm. Supér. (4) 50(3) (2017), .Google Scholar
Dinh, V. D., Dynamics of radial solutions for the focusing fourth-order nonlinear Schrödinger equations, Nonlinearity 34(2) (2021), 776–821.CrossRefGoogle Scholar
Dinh, V. D. and Forcella, L., Blow-up results for systems of nonlinear Schrödinger equations with quadratic interaction, Z. Angew. Math. Phys. 72(178) (2021), .CrossRefGoogle Scholar
Fibich, G., Ilan, B. and Papanicolaou, G., Self-focusing with fourth-order dispersion, SIAM J. Appl. Math. 62(4) (2002), 1437–1462.Google Scholar
Forcella, L., On finite time blow-up for a 3D Davey-Stewartson system, Proc. Amer. Math. Soc. 150(12) (2022), 5421–5432.Google Scholar
Guo, Q., Scattering for the focusing L 2-supercritical and H 2-subcritical biharmonic NLS equations, Comm. Partial Differential Equations 41(2) (2016), 185–207.CrossRefGoogle Scholar
Karpman, V.I., Stabilization of soliton instabilities by higher-order dispersion: Fourth-order non-linear schrödinger-type equations, Phys. Rev. E 53(2) (1996), R1336–R1339.CrossRefGoogle Scholar
Karpman, V.I., and Shagalov, A. G., Stability of solitons described by nonlinear Schrödinger-type equations with higher-order dispersion, Phys. D 144(1-2) (2000), 194–210.CrossRefGoogle Scholar
Martel, Y., Blow-up for the nonlinear Schrödinger equation in nonisotropic spaces, Nonlinear Anal. 28(12) (1997), 1903–1908.CrossRefGoogle Scholar
Miao, C., Xu, G. and Zhao, L., Global well-posedness and scattering for the focusing energy-critical nonlinear Schrödinger equations of fourth order in the radial case, J. Differential Equations 246(9) (2009), 3715–3749.CrossRefGoogle Scholar
Pausader, B., Global well-posedness for energy critical fourth-order Schrödinger equations in the radial case, Dyn. Partial Differ. Equ. 4(3) (2007), 197–225.CrossRefGoogle Scholar
Pausader, B., The cubic fourth-order Schrödinger equation, J. Funct. Anal. 256(8) (2009), 2473–2517.CrossRefGoogle Scholar
Pausader, B., The focusing energy-critical fourth-order Schrödinger equation with radial data, Discrete Contin, Dyn. Syst 24(4) (2009), 1275–1292.Google Scholar
Pausader, B. and Xia, S., Scattering theory for the fourth-order Schrödinger equation in low dimensions, Nonlinearity 26(8) (2013), 2175–2191.CrossRefGoogle Scholar
Strauss, W. A., Existence of solitary waves in higher dimensions, Comm. Math. Phys. 55(2) (1977), 149–162.CrossRefGoogle Scholar