Hostname: page-component-669899f699-chc8l Total loading time: 0 Render date: 2025-05-01T19:14:12.394Z Has data issue: false hasContentIssue false
  • English
  • Français

VARIANTS OF A MULTIPLIER THEOREM OF KISLYAKOV

Part of: Abstract harmonic analysis Harmonic analysis in one variable

Published online by Cambridge University Press:  01 September 2022

Andreas Defant ,
Mieczysław Mastyło Open the ORCID record for Mieczysław Mastyło [Opens in a new window]  and
Antonio Pérez-Hernández Open the ORCID record for Antonio Pérez-Hernández [Opens in a new window]
Andreas Defant
Affiliation:
Institut für Mathematik, Carl von Ossietzky Universität, Postfach 2503 D-26111 Oldenburg, Germany ([email protected])
Mieczysław Mastyło
Affiliation:
Faculty of Mathematics and Computer Science, Adam Mickiewicz University, Poznań, Uniwersytetu Poznańskiego 4, 61-614 Poznań, Poland ([email protected])
Antonio Pérez-Hernández*
Affiliation:
Departamento de Matemática Aplicada I, Escuela Técnica Superior de Ingenieros Industriales, Universidad Nacional de Educación a Distancia (UNED), 28040 Madrid, Spain
*
[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove stronger variants of a multiplier theorem of Kislyakov. The key ingredients are based on ideas of Kislyakov and the Kahane–Salem–Zygmund inequality. As a by-product, we show various multiplier theorems for spaces of trigonometric polynomials on the n-dimensional torus 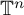$\mathbb {T}^n$ or Boolean cubes 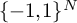$\{-1,1\}^N$. Our more abstract approach based on local Banach space theory has the advantage that it allows to consider more general compact abelian groups instead of only the multidimensional torus. As an application, we show that various recent 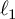$\ell _1$-multiplier theorems for trigonometric polynomials in several variables or ordinary Dirichlet series may be proved without the Kahane–Salem–Zygmund inequality.

Keywords

Fourier analysis on groupsmultipliersSidon setsBoolean functionsmultivariable and Dirichlet polynomials

MSC classification

Primary: 43A46: Special sets (thin sets, Kronecker sets, Helson sets, Ditkin sets, Sidon sets, etc.) 43A75: Analysis on specific compact groups 06E30: Boolean functions 42A16: Fourier coefficients, Fourier series of functions with special properties, special Fourier series
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 23 , Issue 1 , January 2024 , pp. 347 - 377
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Bayart, F., Defant, A., Frerick, L.,Maestre, M. and Sevilla, P., Multipliers of Dirichletseries and monomial series expansion of holomorphic functions in infinitely many variables, Math. Ann. 368(1–2) (2017),837–876.CrossRefGoogle Scholar
Bayart, F., Pellegrino, D. and Seoane-Sepulveda, J., The Bohr radius of the $n$ -dimensional polydisk is equivalent to $\sqrt{\mathit{\log}(n)/ n}$ , Adv. Math. 264 (2014),726–746.CrossRefGoogle Scholar
Bergh, J. and Löfström, J., Interpolation spaces. An introduction. Crundlehren Math. Wiss. 223 (Springer, Berlin, Heidelberg, 1976).Google Scholar
Boas, H. and Khavinson, D., Bohr’s power series theorem in severalvariables, Proc. Amer. Math. Soc. 125 (1997), 2975–2979.CrossRefGoogle Scholar
Bohnenblust, H. F. and Hille, E., On the absolute convergence of Dirichlet series, Ann. of Math. 32(3) (1931), 600–622.CrossRefGoogle Scholar
Bohr, H., A theorem concerning power series, Proc. London Math. Soc. 13(2) (1914), 1–5.CrossRefGoogle Scholar
Bourgain, J., Bounded orthogonal systems and the $\varLambda (p)$ -set problem, Acta Math. 162(3–4) (1989),227–245.CrossRefGoogle Scholar
Defant, A., Frerick, L., Ortega-Cerdá, J., Ounäıes, M. and Seip, K., The Bohnenblust-Hille inequality for homogeneous polynomials is hypercontractive, Ann. of Math. (2) 174(1) (2011), 485–497.CrossRefGoogle Scholar
Defant, A., García, D., Maestre, M. and Sevilla Peris, P., Dirichlet Series and Holomorphic Functions in High Dimensions, New Mathematical Monographs Series. Vol. 37 (Cambridge University Press, Cambridge, 2019).Google Scholar
Defant, A. and Mastyło, M., Aspects of the Kahane–Salem–Zygmund inequalities in Banachspaces, Preprint, 2022.CrossRefGoogle Scholar
Defant, A., Mastyło, M. and Pérez, A., Bohr’s phenomenon for functions on the Boolean cube, J. Funct. Anal. 275(11) (2011), 3115–3147.CrossRefGoogle Scholar
Defant, A., Mastyło, M. and Pérez, A., On the Fourier spectrum of functions on Boolean cubes, Math. Ann. 374(1–2)(2019), 653–680.CrossRefGoogle Scholar
Diestel, J., Jarchow, H. and Tonge, A., Absolutely summing operators, in Cambridge Studies in Advanced Mathematics. Vol 43 (Cambridge University Press, Cambridge, 1995).Google Scholar
Kahane, J.-P., Some Random Series of Functions, 2nd ed., Cambridge Studies in Advanced Mathematics. Vol. 5 (Cambridge University Press, Cambridge, New York, 1985).Google Scholar
Kislyakov, S., Fourier coeffcients of boundary values of analytic functions in the disc and bidisc $\mathrm{II}$ , Tr. Mat. Inst. Steklova 155 (1981), 77–91 (inRussian).Google Scholar
Klimek, M., Metrics associated with extremal plurisubharmonic functions, Proc. Amer. Math. Soc. 123(9) (1995), 2763–2770.CrossRefGoogle Scholar
O’Donnell, R., Analysis of Boolean Functions (Cambridge University Press, New York, 2014).CrossRefGoogle Scholar
Pełczyński, A., $p$ -integral operators commuting with group representation and examples of quasi- $p$ -integral operators which are not $p$ -integral, Studia Math. 33(1) (1969),63–70.CrossRefGoogle Scholar
Pisier, G., Factorization of Linear Operators and Geometry of Banach Spaces, 60 (Amer. Math. Soc., CBMS, Providence, R.I., 1985).Google Scholar
Queffélec, H. and Queffélec, M., Diophantine Approximation and Dirichlet Series, HRI Lecture Notes Series. Vol. 2 (Hindustan Book Agency, New Delhi, 2013).CrossRefGoogle Scholar
Visser, C., A generalization of Tchebychef’s inequality to polynomials in more than one variable, Indagationes Math. 8 (1946), 310–311.Google Scholar