No CrossRef data available.
Published online by Cambridge University Press: 23 October 2018
We consider an extension of the Ramanujan series with a variable  $x$. If we let
$x$. If we let  $x=x_{0}$, we call the resulting series ‘Ramanujan series with the shift
$x=x_{0}$, we call the resulting series ‘Ramanujan series with the shift 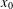 $x_{0}$’. Then we relate these shifted series to some
$x_{0}$’. Then we relate these shifted series to some  $q$-series and solve the case of level
$q$-series and solve the case of level 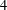 $4$ with the shift
$4$ with the shift  $x_{0}=1/2$. Finally, we indicate a possible way towards proving some patterns observed by the author corresponding to the levels
$x_{0}=1/2$. Finally, we indicate a possible way towards proving some patterns observed by the author corresponding to the levels  $\ell =1,2,3$ and the shift
$\ell =1,2,3$ and the shift 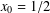 $x_{0}=1/2$.
$x_{0}=1/2$.