Hostname: page-component-669899f699-tzmfd Total loading time: 0 Render date: 2025-04-28T18:31:03.384Z Has data issue: false hasContentIssue false
  • English
  • Français

DISCRETE SETS DEFINABLE IN STRONG EXPANSIONS OF ORDERED ABELIAN GROUPS

Part of: Model theory

Published online by Cambridge University Press:  13 December 2024

ALFRED DOLICH  and
JOHN GOODRICK
ALFRED DOLICH
Affiliation:
DEPARTMENT OF MATHEMATICS AND COMPUTER SCIENCE KINGSBOROUGH COMMUNITY COLLEGE (CUNY) 2001 ORIENTAL BOULEVARD BROOKLYN, NY 11235 USA DEPARTMENT OF MATHEMATICS CUNY GRADUATE CENTER 365 5TH AVENUE NEW YORK, NY 10016 USA E-mail: [email protected]
JOHN GOODRICK*
Affiliation:
DEPARTAMENTO DE MATEMÁTICAS UNIVERSIDAD DE LOS ANDES CARRERA 1 NO. 18A-12 BOGOTÁ 111711 COLOMBIA
*
E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the structure of infinite discrete sets D definable in expansions of ordered Abelian groups whose theories are strong and definably complete, with a particular emphasis on the set 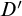$D'$ comprised of differences between successive elements. In particular, if the burden of the structure is at most n, then the result of applying the operation $D \mapsto D'\ n$ times must be a finite set (Theorem 1.1). In the case when the structure is densely ordered and has burden 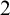$2$, we show that any definable unary discrete set must be definable in some elementary extension of the structure $\langle \mathbb{R}; <, +, \mathbb{Z} \rangle $ (Theorem 1.3).

Keywords

ordered Abelian groupsdp-rankburdendiscrete sets

MSC classification

Primary: 03C45: Classification theory, stability and related concepts
Type
Article
Information
The Journal of Symbolic Logic , Volume 90 , Issue 1 , March 2025 , pp. 423 - 459
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

REFERENCES

Belagradek, O., Peterzil, Y. and Wagner, F., Quasi-o-minimal structures . The Journal of Symbolic Logic, vol. 65 (2000), no. 3, pp. 1115–1132.CrossRefGoogle Scholar
Cluckers, R., Presburger sets and P-minimal fields . The Journal of Symbolic Logic, vol. 68 (2003), no. 1, pp. 153–162.CrossRefGoogle Scholar
Dolich, A. and Goodrick, J., Strong theories of ordered abelian groups . Fundamenta Mathematicae, vol. 236 (2017), pp. 269–296.CrossRefGoogle Scholar
Dolich, A. and Goodrick, J., Topological properties of definable sets in ordered abelian groups of burden 2 . Mathematical Logic Quarterly, vol. 69 (2023), no. 2, pp. 147–164.CrossRefGoogle Scholar
Dolich, A., Goodrick, J. and Lippel, D., Dp-minimal theories: Basic facts and examples . Notre Dame Journal of Formal Logic, vol. 52 (2011), no. 3, pp. 267–288.CrossRefGoogle Scholar
van den Dries, L., O-minimal structures and real analytic geometry , Current Developments in Mathematics, International Press, Cambridge, 1998, pp. 105–152.Google Scholar
Fujita, M., Locally o-minimal structures with tame topological properties . The Journal of Symbolic Logic, vol. 88 (2021), no. 1, pp. 219–241.CrossRefGoogle Scholar
Fujita, M., Simple product and locally o-minimal theories, preprint, 2021, arXiv:2110.11596v1.Google Scholar
Goodrick, J., A monotonicity theorem for dp-minimal densely ordered groups . The Journal of Symbolic Logic, vol. 75 (2010), no. 1, pp. 221–238.CrossRefGoogle Scholar
Kaplan, I., Onshuus, A. and Usvyatsov, A., Additivity of the dp-rank . Transactions of the American Mathematical Society, vol. 365 (2013), pp. 5783–5804.CrossRefGoogle Scholar
Kawakami, T., Takeuchi, K., Tanaka, H. and Tsuboi, A., Locally o-minimal structures . Journal of the Matematical Society of Japan, vol. 64 (2012), pp. 783–797.Google Scholar
Levi, F. W., Ordered groups . Proceedings of the Indian Academy of Sciences—Section A, vol. 16 (1942), pp. 256–263.CrossRefGoogle Scholar
Miller, C., Expansions of dense linear orders with the intermediate value property . The Journal of Symbolic Logic, vol. 66 (2001), pp. 1783–1790.CrossRefGoogle Scholar
Onshuus, A. and Usvyatsov, A., On dp-minimality, strong dependence, and weight . The Journal of Symbolic Logic, vol. 76 (2011), no. 3, pp. 737–758. Available on the second author’s website.CrossRefGoogle Scholar
Shelah, S., Strongly dependent theories . Israel Journal of Mathematics, vol. 204 (2014), no. 1, pp. 1–83.CrossRefGoogle Scholar
Simon, P., On dp-minimal ordered structures . The Journal of Symbolic Logic, vol. 76 (2011), no. 2, pp. 448–460.CrossRefGoogle Scholar
Simon, P., A Guide to NIP Theories, Cambridge University Press, Cambridge, 2015.CrossRefGoogle Scholar
Touchard, P., Burden in Henselian valued fields . Annals of Pure and Applied Logic, vol. 174 (2023), no. 10, Article no. 103318.CrossRefGoogle Scholar