Hostname: page-component-669899f699-tzmfd Total loading time: 0 Render date: 2025-04-27T17:14:07.476Z Has data issue: false hasContentIssue false
  • English
  • Français

A necessary condition for breakeven in dipole-confined plasmas

Published online by Cambridge University Press:  22 October 2024

A. Di Vita Open the ORCID record for A. Di Vita [Opens in a new window]
A. Di Vita*
Affiliation:
D.I.C.C.A., Università di Genova, Via Montallegro 1, 16145 Genova, Italy
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We have derived a necessary condition for the achievement of breakeven in axisymmetric plasmas with zero toroidal field and confined by a dipole magnetic field (B. Lehnert, Nature, vol. 181, 1958, p. 4605; A. Hasegawa, Comments Plasma Phys. Control. Fusion, vol. 11, no. 3, 1987). Excellent MHD stability, high values of $\beta$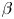 (up to $26$ 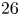%) and good confinement properties awaken the interest of private investors after years of neglect due to lack of public funding and competing alternative lines of research like the tokamak. Starting from a requirement of self-consistency between the balances of momentum and energy in a dipole-confined, two-species plasma and assuming a Maxwellian distribution function for ions and electrons, we derive a necessary condition for breakeven. This condition is more stringent than the Lawson criterion because of the lack of a stabilizing toroidal field. For a given current flowing across the toroidal coil internal to the plasma, the crucial factor at stake is the ratio between the radius of the main toroidal coil and the radius of the vacuum chamber.

Keywords

fusion plasmaplasma confinementdipole-confined plasma
Type
Research Article
Information
Journal of Plasma Physics , Volume 90 , Issue 5 , October 2024 , 905900502
Check for updates
Copyright
Copyright © The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Anderson, O.A., Birdsall, D.H., Hartman, C.W., Lauer, E.J. & Furth, H.P. 1968 Plasma confinement in the levitron. In Proceedings of the 3rd IAEA International Conference on Plasma Physics and Controlled Nuclear Fusion Research, Novosibirsk, 1–7 August 1968.Google Scholar
Berry, T., Mataira-Cole, R. & Simpson, T. 2023 Advancements in the levitated dipole reactor at OpenStar technologies. In 65th Annual Meeting of the APS Division of Plasma Physics, October 30–November 3 2023, Denver, Colorado, USA.Google Scholar
Binderbauer, M.W., et al. 2015 A high performance field-reversed configuration. Phys. Plasmas 22, 056110.CrossRefGoogle Scholar
Boxer, A.C., Bergmann, R., Ellsworth, J.L., Garnier, D.T., Kesner, J., Mauel, M.E. & Woskov, P. 2010 Turbulent inward pinch of plasma confined by a levitated dipole magnet. Nat. Phys. 6, 207–212.CrossRefGoogle Scholar
Breton, C. & Ya'akobi, B. 1973 Plasma confinement in a high density toroidal hard-core device. Plasma Phys. 15, 1067–1082.CrossRefGoogle Scholar
Connor, J.W. & Taylor, J.B. 1977 Scaling laws for plasma confinement. Nucl. Fusion 17 (5), 1047.CrossRefGoogle Scholar
Davis, M.S. 2013 Pressure profiles of plasmas confined in the field of a dipole magnet. PhD thesis, Columbia University.Google Scholar
Di Vita, A. 2009 Hot spots and filaments in the pinch of a plasma focus: a unified approach. Eur. Phys. J. D 54, 451–461.CrossRefGoogle Scholar
Di Vita, A. 2022 Non-Equilibrium Thermodynamics. Springer.CrossRefGoogle Scholar
Dolan, T. 1982 Fusion Research. Pergamon.Google Scholar
Edlington, T., Fletcher, W.H.W., Riviere, A.C. & Todd, T.N. 1980 Particle confinement scaling experiments in the Culham Levitron. Nucl. Fusion 20, 825–831.CrossRefGoogle Scholar
Elio, F. 2014 Revisiting the poloidal magnetic confinement. Fusion Engng Design 89, 806–811.CrossRefGoogle Scholar
Elsgolts, I.V. 1981 Differential Equations and Variational Calculus. Mir Moscow.Google Scholar
Freeman, R., Okabayashi, M., Pacher, G. & Yoshikawa, S. 1969 Plasma containment in the Princeton SPHERATOR using a supported superconducting ring. Phys. Rev. Lett. 23 (14), 756–760.CrossRefGoogle Scholar
Freidberg, J. 2014 Ideal MHD. Cambridge University Press.CrossRefGoogle Scholar
Garnier, D.T., et al. 2006 Design and initial operation of the LDX facility. Fusion Engng Design 81, 2371–2380.CrossRefGoogle Scholar
Garnier, D.T., Boxer, A.C., Ellsworth, J.L., Kesner, J. & Mauel, M.E. 2009 Confinement improvement with magnetic levitation of a superconducting dipole. Nucl. Fusion 49 (5), 055023.CrossRefGoogle Scholar
Garnier, D.T., Kesner, J. & Mauel, M.E. 1999 Magnetohydrodynamic stability in a levitated dipole. Phys. Plasmas 6, 3431.CrossRefGoogle Scholar
Greenwald, M. 2002 Density limits in toroidal plasmas. Plasma Phys. Control. Fusion 44, R27–R80.CrossRefGoogle Scholar
Hasegawa, A. 1987 A dipole field fusion reactor. Comments Plasma Phys. Control. Fusion 11 (3), 147–151.Google Scholar
Hermann, F. 1986 Simple examples of the theorem of minimum entropy production. Eur. J. Phys. 7, 130–131.CrossRefGoogle Scholar
Kesner, J. 1997 Stability of electrostatic modes in a levitated dipole. Phys. Plasmas 4 (2), 419–422.CrossRefGoogle Scholar
Kesner, J., Garnier, D.T., Hansen, A., Mauel, M. & Bromberg, L. 2004 Helium catalyzed D-D fusion in a levitated dipole in a Z-pinch. Nucl. Fusion 44 (1), 193–203.CrossRefGoogle Scholar
Kesner, J. & Hastie, R.J. 2002 Electrostatic drift modes in a closed field line configuration. Phys. Plasmas 9 (2), 395–400.CrossRefGoogle Scholar
Kesner, J. & Mauel, M.E. 1997 Plasma confinement in a levitated magnetic dipole. Plasma Phys. Rep. 23 (9), 742–750.Google Scholar
Kesner, J. & Mauel, M.E. 2013 Final report: levitated dipole experiment. Available at: https://www.osti.gov/servlets/purl/1095287.CrossRefGoogle Scholar
Kobayashi, S., Rogers, B.N. & Dorland, W. 2010 Particle pinch in gyrokinetic simulations of closed field-line systems. Phys. Rev. Lett. 105, 235004.CrossRefGoogle ScholarPubMed
Krashenninikov, S.I., Catto, P.J. & Hazeltine, R.D. 1999 Magnetic dipole equilibrium solution at finite plasma pressure. Phys. Rev. Lett. 82 (13), 2689–2692.CrossRefGoogle Scholar
Lao, L.L., Hirshman, S.P. & Wieland, R.M. 1981 Variational moment solutions to the Grad-Shafranov equation. Oak Ridge National Laboratory ORNL/TM-7616.CrossRefGoogle Scholar
Lawson, J.D. 1957 Some criteria for a power producing thermonuclear reactor. Proc. Phys. Soc. B 70, 6–10.CrossRefGoogle Scholar
Lehnert, B. 1958 Confinement of charged particles by a magnetic field. Nature 181 (4605), 331–332.CrossRefGoogle Scholar
Lehnert, B. 1968 a Plasma confinement in ring-current configurations. Plasma Phys. 10, 263–279.CrossRefGoogle Scholar
Lehnert, B. 1968 b On the possibilities of ring-current configurations as a fusion device. Plasma Phys. 10, 281–289.CrossRefGoogle Scholar
Mauel, M. 2008 Improved confinement during magnetic levitation in LDX. In Bulletin of the American Physical Society (Proceedings of the 50th Annual Meeting of the APS Division of Plasma Physics, Dallas, USA), 2008 November, vol. 53, no. 14.Google Scholar
Perkins, F.W., et al. 1993 Nondimensional transport scaling in the tokamak fusion test reactor: is tokamak transport Bohm or gyro-Bohm? Phys. Fluids B 5, 477–498.CrossRefGoogle Scholar
Rogister, A. & Li, D. 1992 Kinetic and transport theories of turbulent, axisymmetric, low-collisionality plasmas and turbulence constraints. Phys. Fluids B 4 (4), 804–830.CrossRefGoogle Scholar
Romanelli, M. & Orsitto, F.P. 2021 On similarity scaling of tokamak fusion plasmas with different aspect ratio. Plasma Phys. Control. Fusion 63, 125004.CrossRefGoogle Scholar
Saitoh, H., et al. 2010 High-beta plasma confinement and inward particle diffusion in the magnetospheric device RT-1. In EXC/9-4Rb 23rd IAEA FEC, 11–16 October 2010. Available at: https://www-internal.psfc.mit.edu/ldx/reports/ICC1-1Ra_garnier_LDX_RT1.pdf.Google Scholar
Saitoh, H., et al. 2011 High-$\beta$ plasma formation and observation of peaked density profile in RT-1. Nucl. Fusion 51, 063034.CrossRefGoogle Scholar
Simakov, A.N. 2001 Plasma stability in a dipole magnetic field. PhD thesis, MIT, Boston.Google Scholar
Simakov, A.N., Catto, P.J., Krashenninikov, N.S. & Ramos, J.J. 2000 Ballooning stability of a point dipole equilibrium. Phys. Plasmas 7, 2526–2529.CrossRefGoogle Scholar
Skellett, S. 1975 The Culham superconducting levitron. Cryogenics 15 (10), 563–568.CrossRefGoogle Scholar
Taylor, J.B. 1997 Turbulence in two-dimensional plasmas and fluids. Plasma Phys. Control. Fusion 39 (5A), A1.CrossRefGoogle Scholar
Teller, E., Glass, A.J., Fowler, T.K., Hasegawa, A. & Santarius, J.F. 1992 Space propulsion by fusion in a magnetic dipole. Fusion Technol. 22 (1), 82–97.CrossRefGoogle Scholar
Yoshida, Z., Saitoh, H., Yano, Y., Mikami, H., Kasaoka, N., Sakamoto, W., Morikawa, J., Furukawa, M. & Mahajan, S.M. 2013 Self-organized confinement by magnetic dipole: recent results from RT-1 and theoretical modeling. Plasma Phys. Control. Fusion 55, 014018.CrossRefGoogle Scholar