Hostname: page-component-669899f699-b58lm Total loading time: 0 Render date: 2025-04-27T13:53:23.779Z Has data issue: false hasContentIssue false
  • English
  • Français

A geometric p-adic Simpson correspondence in rank one

Part of: Families, fibrations Arithmetic problems. Diophantine geometry

Published online by Cambridge University Press:  21 May 2024

Ben Heuer Open the ORCID record for Ben Heuer [Opens in a new window]
Ben Heuer*
Affiliation:
Institut für Mathematik, Johann Wolfgang Goethe-Universität, Robert-Mayer-Str. 6-8, 60325 Frankfurt am Main, Germany [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For any smooth proper rigid space 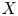$X$ over a complete algebraically closed extension $K$ of 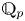$\mathbb {Q}_p$ we give a geometrisation of the 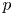$p$-adic Simpson correspondence of rank one in terms of analytic moduli spaces: the 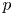$p$-adic character variety is canonically an étale twist of the moduli space of topologically torsion Higgs line bundles over the Hitchin base. This also eliminates the choice of an exponential. The key idea is to relate both sides to moduli spaces of 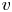$v$-line bundles. As an application, we study a major open question in 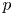$p$-adic non-abelian Hodge theory raised by Faltings, namely which Higgs bundles correspond to continuous representations under the 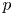$p$-adic Simpson correspondence. We answer this question in rank one by describing the essential image of the continuous characters 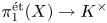$\pi ^{{\mathrm {\acute {e}t}}}_1(X)\to K^\times$ in terms of moduli spaces: for projective 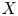$X$ over 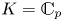$K=\mathbb {C}_p$, it is given by Higgs line bundles with vanishing Chern classes like in complex geometry. However, in general, the correct condition is the strictly stronger assumption that the underlying line bundle is a topologically torsion element in the topological group $\operatorname {Pic}(X)$.

Keywords

p-adic Simpson correspondencep-adic non-abelian Hodge theorymoduli spacev-vector bundle

MSC classification

Primary: 14D22: Fine and coarse moduli spaces
Secondary: 14G22: Rigid analytic geometry 14D10: Arithmetic ground fields (finite, local, global)
Type
Research Article
Information
Compositio Mathematica , Volume 160 , Issue 7 , July 2024 , pp. 1433 - 1466
Check for updates
Copyright
© 2024 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Abbes, A., Gros, M. and Tsuji, T., The p-adic Simpson correspondence, Annals of Mathematics Studies, vol. 193 (Princeton University Press, Princeton, NJ, 2016).10.1515/9781400881239CrossRefGoogle Scholar
Bhatt, B. and Scholze, P., The pro-étale topology for schemes, Astérisque 369 (2015), 99–201.Google Scholar
Deninger, C. and Werner, A., Line bundles and p-adic characters, in Number fields and function fields—two parallel worlds, Progress in Mathematics, vol. 239 (Birkhäuser, Boston, MA, 2005), 101–131.10.1007/0-8176-4447-4_7CrossRefGoogle Scholar
Deninger, C. and Werner, A., Vector bundles on p-adic curves and parallel transport, Ann. Sci. Éc. Norm. Supér. (4) 38 (2005), 553–597.10.1016/j.ansens.2005.05.002CrossRefGoogle Scholar
Deninger, C. and Werner, A., Parallel transport for vector bundles on p-adic varieties, J. Algebraic Geom. 29 (2020), 1–52.10.1090/jag/747CrossRefGoogle Scholar
Faltings, G., A p-adic Simpson correspondence, Adv. Math. 198 (2005), 847–862.10.1016/j.aim.2005.05.026CrossRefGoogle Scholar
Fargues, L., Groupes analytiques rigides p-divisibles, Math. Ann. 374 (2019), 723–791.10.1007/s00208-018-1782-9CrossRefGoogle Scholar
Fontaine, J.-M., Presque $C_p$-représentations, Doc. Math., Extra Vol., Kazuya Kato's Fiftieth Birthday (2003), 285–385.Google Scholar
Groechenig, M., Moduli of flat connections in positive characteristic, Math. Res. Lett. 23 (2016), 989–1047.10.4310/MRL.2016.v23.n4.a3CrossRefGoogle Scholar
Guo, H., Hodge–Tate decomposition for non-smooth spaces, J. Eur. Math. Soc. 25 (2023), 1553–1625.10.4171/jems/1222CrossRefGoogle Scholar
Hansen, D. and Li, S., Line bundles on rigid varieties and Hodge symmetry, Math. Z. 296 (2020), 1777–1786.10.1007/s00209-020-02535-3CrossRefGoogle Scholar
Hartl, U. and Lütkebohmert, W., On rigid-analytic Picard varieties, J. Reine Angew. Math. 528 (2000), 101–148.Google Scholar
Heuer, B., Diamantine Picard functors of rigid spaces, Preprint (2021), arXiv:2103.16557.Google Scholar
Heuer, B., Line bundles on perfectoid covers: case of good reduction, Preprint (2021), arXiv:2105.05230.Google Scholar
Heuer, B., Pro-étale uniformisation of abelian varieties, Preprint (2021), arXiv:2105.12604.Google Scholar
Heuer, B., $G$-torsors on perfectoid spaces, Preprint (2022), arXiv:2207.07623.Google Scholar
Heuer, B., Line bundles on rigid spaces in the $v$-topology, Forum Math. Sigma 10 (2022), e82.10.1017/fms.2022.72CrossRefGoogle Scholar
Heuer, B., Moduli spaces in $p$-adic non-abelian Hodge theory, Preprint (2022), arXiv:2207.13819.Google Scholar
Heuer, B., A $p$-adic Simpson correspondence for smooth proper rigid spaces, Preprint (2023), arXiv:2307.01303.Google Scholar
Heuer, B., Mann, L. and Werner, A., The $p$-adic Corlette–Simpson correspondence for abeloids, Math. Ann. 385 (2023), 1639–1676.10.1007/s00208-022-02371-2CrossRefGoogle Scholar
Kedlaya, K. S. and Liu, R., Relative $p$-adic Hodge theory, II: imperfect period rings, Preprint (2016), arXiv:1602.06899.Google Scholar
Lütkebohmert, W., Rigid geometry of curves and their Jacobians, in Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge, A Series of Modern Surveys in Mathematics, vol. 61 (Springer, Cham, 2016).Google Scholar
Mann, L. and Werner, A., Local systems on diamonds and $p$-adic vector bundles, Int. Math. Res. Not. IMRN 2023 (2023), 12785–12850.10.1093/imrn/rnac182CrossRefGoogle Scholar
Morrow, M. and Tsuji, T., Generalised representations as q-connections in integral $p$-adic Hodge theory, Preprint (2021), arXiv:2010.04059.Google Scholar
Neukirch, J., Schmidt, A. and Wingberg, K., Cohomology of number fields, second edition, Grundlehren der Mathematischen Wissenschaften, vol. 323 (Springer, Berlin, 2008).10.1007/978-3-540-37889-1CrossRefGoogle Scholar
Scholze, P., Perfectoid spaces, Publ. Math. Inst. Hautes Études Sci. 116 (2012), 245–313.10.1007/s10240-012-0042-xCrossRefGoogle Scholar
Scholze, P., $p$-adic Hodge theory for rigid-analytic varieties, Forum Math. Pi 1 (2013), e1.10.1017/fmp.2013.1CrossRefGoogle Scholar
Scholze, P., Étale cohomology of diamonds, Preprint (2018), arXiv:1709.07343.Google Scholar
Scholze, P. and Weinstein, J., Moduli of $p$-divisible groups, Camb. J. Math. 1 (2013), 145–237.10.4310/CJM.2013.v1.n2.a1CrossRefGoogle Scholar
Scholze, P. and Weinstein, J., Berkeley lectures on p-adic geometry, Annals of Mathematics Studies (Princeton University Press, Princeton, NJ, 2020).Google Scholar
Grothendieck, A. and Raynaud, M., Revêtements étales et groupe fondamental (SGA 1), Documents Mathématiques (Paris), vol. 3, Séminaire de géométrie algébrique du Bois Marie 1960–61 (Société Mathématique de France, Paris, 2003).Google Scholar
Simpson, C., Higgs bundles and local systems, Inst. Hautes Études Sci. Publ. Math. 75 (1992), 5–95.10.1007/BF02699491CrossRefGoogle Scholar
Simpson, C., Subspaces of moduli spaces of rank one local systems, Ann. Sci. Éc. Norm. Supér. (4) 26 (1993), 361–401.10.24033/asens.1675CrossRefGoogle Scholar
Simpson, C., Moduli of representations of the fundamental group of a smooth projective variety. II, Inst. Hautes Études Sci. Publ. Math. (1994) 80 (1995), 5–79.10.1007/BF02698895CrossRefGoogle Scholar
Simpson, C., The Hodge filtration on nonabelian cohomology, in Algebraic geometry Santa Cruz 1995, Proceedings of Symposia in Pure Mathematics, vol. 62 (American Mathematical Society, Providence, RI, 1997), 217–281.10.1090/pspum/062.2/1492538CrossRefGoogle Scholar
Song, Z., Rigid analytic $p$-adic Simpson correspondence for line bundles, Commun. Math. Stat. 10 (2022), 739–756.10.1007/s40304-021-00256-5CrossRefGoogle Scholar
Tate, J. T., p-divisible groups, in Proceedings of a conference on local fields (Driebergen, 1966) (Springer, Berlin, 1967), 158–183.10.1007/978-3-642-87942-5_12CrossRefGoogle Scholar
Wang, Y., A $p$-adic Simpson correspondence for rigid analytic varieties, Algebra Number Theory 17 (2023), 1453–1499.10.2140/ant.2023.17.1453CrossRefGoogle Scholar
Würthen, M., Vector bundles with numerically flat reduction on rigid analytic varieties and $p$-adic local systems, Int. Math. Res. Not. IMRN 2023 (2023), 4004–4045.10.1093/imrn/rnab214CrossRefGoogle Scholar
Xu, D., Parallel transport for Higgs bundles over $p$-adic curves, Preprint (2022), arXiv:2201.06697, with an Appendix by Daxin Xu and Tongmu He.Google Scholar