Hostname: page-component-669899f699-ggqkh Total loading time: 0 Render date: 2025-04-30T04:22:40.296Z Has data issue: false hasContentIssue false
  • English
  • Français

Twisted Whittaker category on affine flags and the category of representations of the mixed quantum group

Part of: Families, fibrations Lie algebras and Lie superalgebras

Published online by Cambridge University Press:  13 May 2024

Ruotao Yang
Ruotao Yang*
Affiliation:
Igor Krichever Center for Advanced Studies, Skolkovo Institute of Science and Technology, Moscow 121205, Russia [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 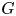$G$ be a reductive group, and let 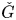$\check {G}$ be its Langlands dual group. Arkhipov and Bezrukavnikov proved that the Whittaker category on the affine flags 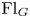${\operatorname {Fl}}_G$ is equivalent to the category of 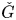$\check {G}$-equivariant quasi-coherent sheaves on the Springer resolution of the nilpotent cone. This paper proves this theorem in the quantum case. We show that the twisted Whittaker category on ${\operatorname {Fl}}_G$ and the category of representations of the mixed quantum group are equivalent. In particular, we prove that the quantum category 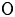$\mathsf {O}$ is equivalent to the twisted Whittaker category on 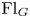${\operatorname {Fl}}_G$ in the generic case. The strong version of our main theorem claims a motivic equivalence between the Whittaker category on ${\operatorname {Fl}}_G$ and a factorization module category, which holds in the de Rham setting, the Betti setting, and the 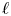$\ell$-adic setting.

Keywords

Whittaker D-modulesaffine flagsquantum groups

MSC classification

Primary: 14D24: Geometric Langlands program
Secondary: 17B37: Quantum groups (quantized enveloping algebras) and related deformations
Type
Research Article
Information
Compositio Mathematica , Volume 160 , Issue 6 , June 2024 , pp. 1349 - 1417
Check for updates
Copyright
© 2024 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Arkhipov, S. and Bezrukavnikov, R., Perverse sheaves on affine flags and Langlands dual group, Israel J. Math. 170 (2009), 135–183.CrossRefGoogle Scholar
Beauville, A. and Laszlo, Y., Un lemme de descente, C. R. Math. Acad. Sci. Ser. I 320 (1995), 335–340.Google Scholar
Beilinson, A. and Drinfeld, V., Quantization of Hitchin's fibration and Langland's program, in Algebraic and geometric methods in mathematical physics, Mathematical Physics Studies, vol. 19, eds de Monvel, A.B. and Marchenko, V. (Springer, 1996).Google Scholar
Beilinson, A. and Drinfeld, V., Chiral algebras, AMS Colloquium Publications, vol. 51 (American Mathematical Society, 2004).CrossRefGoogle Scholar
Beligiannis, A. and Reiten, I., Homological and homotopical aspects of torsion theories, Mem. Amer. Math. Soc. 188 (2007), Theorem III.2.3.Google Scholar
Beraldo, D., Loop group actions on categories and Whittaker invariants, Adv. Math. 322 (2017), 565–636.CrossRefGoogle Scholar
Bernstein, I. N., Gelfand, I. M. and Gelfand, S. I., Structure of representations generated by highest weights, Funktsional. Anal. i Prilozhen. 5 (1971), 1–9; English transl., Funct. Anal. Appl. 5 (1971), 1–8.CrossRefGoogle Scholar
Bezrukavnikov, R., Cohomology of tilting modules over quantum groups and t-structures on derived categories of coherent sheaves, Inv. Math. 166 (2006), 327–357.CrossRefGoogle Scholar
Bezrukavnikov, R., On two geometric realizations of an affine Hecke algebra, Publ. Math. Inst. Hautes Études Sci. 123 (2016), 1–67.CrossRefGoogle Scholar
Bezrukavnikov, R., Finkelberg, M. and Schechtman, V., Factorizable sheaves and quantum groups, Lecture Notes in Mathematics, vol. 1691 (Springer, 1998).CrossRefGoogle Scholar
Borel, A., Admissible representations of a semi-simple group over a local field with vector fixed under an Iwahori subgroup, Invent. Math. 35 (1976), 233–260.CrossRefGoogle Scholar
Braverman, A., Finkelberg, M., Gaitsgory, D. and Mirković, I., Intersection cohomology of Drinfeld's compactifications, Selecta Math. (N.S.) 8 (2002), 381–418.CrossRefGoogle Scholar
Braverman, A., Finkelberg, M. and Travkin, R., Gaiotto conjecture for ${\operatorname {Rep}}_{\rm q}({\mathop {\operatorname {\rm GL}}}(N-1|N))$, Pure Appl. Math. Q. (150th birthday of Kazhdan-Lusztig volume), to appear. Preprint (2021), arXiv:2107.02653.Google Scholar
Braverman, A. and Gaitsgory, D., Geometric Eisenstein series, Invent. Math. 150 (2002), 287–384.CrossRefGoogle Scholar
Campbell, J., A resolution of singularities for Drinfeld's compactification by stable maps, J. Algebraic Geom. 28 (2019), 153–167.CrossRefGoogle Scholar
Campbell, J., Dhillon, G. and Raskin, S., Fundamental local equivalences in quantum geometric Langlands, Compos. Math. 157 (2021), 2699–2732.CrossRefGoogle Scholar
Chen, L. and Fu, Y., An extension of the Kazhdan–Lusztig equivalence, Preprint (2021), arXiv:2111.14606.Google Scholar
Deligne, P., SGA 4 1/2–Cohomologie étale, Lecture Notes in Mathematics, vol. 569 (Springer, 1977).Google Scholar
Drinfeld, V. and Gaitsgory, D., On a theorem of Braden, Transform. Groups 19 (2014), 313–358. arXiv:1308.3786 [math.AG]CrossRefGoogle Scholar
Finkelberg, M. and Mirković, I., Semiinfinite flags. I. Case of global curve ${\mathbb {P}}^1$, in Differential topology, infinite-dimensional Lie algebras, and applications, American Mathematical Society Translations: Series 2, vol. 194 (American Mathematical Society, 1999), 81–112.Google Scholar
Frenkel, E., Gaitsgory, D. and Vilonen, K., Whittaker patterns in the geometry of moduli spaces of bundles on curves, Ann. of Math. (2) 153 (2001), 699–748.CrossRefGoogle Scholar
Gaitsgory, D., Twisted Whittaker model and factorization algebras, Selecta Math. (N.S.) 13 (2008), 617.CrossRefGoogle Scholar
Gaitsgory, D., Contractibility of the space of rational maps, Invent. Math. 191 (2013), 91–196.CrossRefGoogle Scholar
Gaitsgory, D., Quantum Langlands correspondence, Preprint (2016), arXiv:1601.05279 [math.AG].Google Scholar
Gaitsgory, D., The semi-infinite intersection cohomology sheaf, Adv. Math. 327 (2018), 789–868. arXiv:1703.04199 [math.AG].CrossRefGoogle Scholar
Gaitsgory, D., Winter school on local geometric Langlands theory: program, Preprint (2018), https://lysenko.perso.math.cnrs.fr/Notes_talks_winter2018/GL-1(Dennis).pdf.Google Scholar
Gaitsgory, D., The local and global versions of the Whittaker category, Pure Appl. Math. Q. 16 (2020), 775–904.CrossRefGoogle Scholar
Gaitsgory, D., A conjectural extension of the Kazhdan–Lusztig equivalence, Publ. Res. Inst. Math. Sci. 57 (2021), 1227–1376.CrossRefGoogle Scholar
Gaitsgory, D., On factorization algebras arising in the quantum geometric Langlands theory, Adv. Math. 391 (2021), 107962.CrossRefGoogle Scholar
Gaitsgory, D., The semi-infinite intersection cohomology sheaf-II: the Ran space version, in Representation theory and algebraic geometry (Springer, 2022), 151–265.Google Scholar
Gaitsgory, D. and Lysenko, S., Parameters and duality for the twisted geometric Langlands theory, Selecta Math. (N.S.) 24 (2018), 227–301.CrossRefGoogle Scholar
Gaitsgory, D. and Lysenko, S., Twisted Whittaker category and quantum groups: the ‘small’ FLE, Preprint (2019), arXiv:1903.02279 [math.AG].Google Scholar
Gaitsgory, D. and Nadler, D., Spherical varieties and Langlands duality, Mosc. Math. J. 10 (2010), 65–137.CrossRefGoogle Scholar
Gaitsgory, D. and Rozenblyum, N., A study in derived algebraic geometry, Vol. 1 (American Mathematical Society, 2017).Google Scholar
Gaitsgory, D. and Rozenblyum, N., A study in derived algebraic geometry, Vol. 2 (American Mathematical Society, 2017).Google Scholar
Kac, V. G., Infinite-dimensional Lie algebras (Cambridge University Press, 1990).CrossRefGoogle Scholar
Kazhdan, D. and Lusztig, G., Tensor structures arising from affine Lie algebras. I, J. Amer. Math. Soc. 6 (1993), 905–1011.CrossRefGoogle Scholar
Kazhdan, D. and Lusztig, G., Tensor structures arising from affine Lie algebras. II, J. Amer. Math. Soc. 7 (1994), 335–453.CrossRefGoogle Scholar
Lurie, J., Higher topos theory (Princeton University Press, 2009).CrossRefGoogle Scholar
Lurie, J., Higher algebra, Preprint (2017), https://www.math.ias.edu/~lurie/papers/HA.pdf.Google Scholar
Lusztig, G., Introduction to quantum groups (Springer, 2010).CrossRefGoogle Scholar
Lusztig, G. and Yun, Z., Endoscopy for Hecke categories, character sheaves and representations, Forum Math. Pi. 8 (2020), e12.CrossRefGoogle Scholar
Raskin, S., Chiral principal series categories, Doctoral dissertation, Harvard University (2014).Google Scholar
Raskin, S., W-algebras and Whittaker categories, Selecta Math. (N.S.) 27 (2021), 3.CrossRefGoogle Scholar
Raskin, S., D-modules on infinite dimensional varieties, Preprint, https://gauss.math.yale.edu/~sr2532/dmod.pdf.Google Scholar
Yang, R., A resolution of singularities of Drinfeld compactification with an Iwahori structure, Preprint (2021), arXiv:2104.09862 [math.AG].Google Scholar
Zhao, Y., Tame twistings and $\Theta$-data, Preprint (2020), arXiv:2004.09671 [math.AG].Google Scholar
Zhu, X., An introduction to affine Grassmannians and the geometric Satake equivalence, in Geometry of moduli spaces and representation theory, IAS/Park City Mathematics Series, vol. 24 (American Mathematical Society, 2016).Google Scholar