Hostname: page-component-669899f699-tpknm Total loading time: 0 Render date: 2025-04-30T05:27:03.272Z Has data issue: false hasContentIssue false
  • English
  • Français

Improved algebraic fibrings

Part of: Special aspects of infinite or finite groups

Published online by Cambridge University Press:  13 September 2024

Sam P. Fisher Open the ORCID record for Sam P. Fisher [Opens in a new window]
Sam P. Fisher*
Affiliation:
Mathematical Institute, University of Oxford, Oxford, OX2 6GG, UK [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that a virtually residually finite rationally solvable (RFRS) group $G$ of type 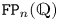$\mathtt {FP}_n(\mathbb {Q})$ virtually algebraically fibres with kernel of type 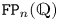$\mathtt {FP}_n(\mathbb {Q})$ if and only if the first 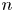$n$ 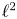$\ell ^2$-Betti numbers of 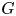$G$ vanish, that is, 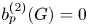$b_p^{(2)}(G) = 0$ for 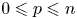$0 \leqslant p \leqslant n$. This confirms a conjecture of Kielak. We also offer a variant of this result over other fields, in particular in positive characteristic. As an application of the main result, we show that amenable virtually RFRS groups of type $\mathtt {FP}(\mathbb {Q})$ are virtually Abelian. It then follows that if 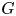$G$ is a virtually RFRS group of type 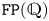$\mathtt {FP}(\mathbb {Q})$ such that 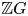$\mathbb {Z} G$ is Noetherian, then 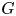$G$ is virtually Abelian. This confirms a conjecture of Baer for the class of virtually RFRS groups of type 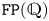$\mathtt {FP}(\mathbb {Q})$, which includes (for instance) the class of virtually compact special groups.

Keywords

RFRS groupsalgebraic fibringfiniteness propertiesHughes-free division rings

MSC classification

Primary: 20F65: Geometric group theory
Type
Research Article
Information
Compositio Mathematica , Volume 160 , Issue 9 , September 2024 , pp. 2203 - 2227
Check for updates
Copyright
© The Author(s), 2024. The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Agol, I., Criteria for virtual fibering, J. Topol. 1 (2008), 269–284.CrossRefGoogle Scholar
Agol, I., The virtual Haken conjecture, Doc. Math. 18 (2013), 1045–1087, with an appendix by I. Agol, Daniel Groves, and Jason Manning.CrossRefGoogle Scholar
Bartholdi, L., Amenability of groups is characterized by Myhill's theorem, J. Eur. Math. Soc. (JEMS) 21 (2019), 3191–3197, with an appendix by Dawid Kielak.CrossRefGoogle Scholar
Bergeron, N., Haglund, F. and Wise, D. T., Hyperplane sections in arithmetic hyperbolic manifolds, J. Lond. Math. Soc. (2) 83 (2011), 431–448.CrossRefGoogle Scholar
Bieri, R., Normal subgroups in duality groups and in groups of cohomological dimension $2$, J. Pure Appl. Algebra 7 (1976), 35–51.CrossRefGoogle Scholar
Bieri, R., Deficiency and the geometric invariants of a group, J. Pure Appl. Algebra 208 (2007), 951–959, with an appendix by Pascal Schweitzer.CrossRefGoogle Scholar
Bieri, R. and Eckmann, B., Finiteness properties of duality groups, Comment. Math. Helv. 49 (1974), 74–83.CrossRefGoogle Scholar
Bieri, R., Neumann, W. D. and Strebel, R., A geometric invariant of discrete groups, Invent. Math. 90 (1987), 451–477.CrossRefGoogle Scholar
Bieri, R. and Renz, B., Valuations on free resolutions and higher geometric invariants of groups, Comment. Math. Helv. 63 (1988), 464–497.CrossRefGoogle Scholar
Brady, N., Branched coverings of cubical complexes and subgroups of hyperbolic groups, J. Lond. Math. Soc. (2) 60 (1999), 461–480.CrossRefGoogle Scholar
Bridson, M. R. and Haefliger, A., Metric spaces of non-positive curvature, Grundlehren der mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences], vol. 319 (Springer, Berlin, 1999).CrossRefGoogle Scholar
Brown, K. S., Cohomology of groups, Graduate Texts in Mathematics, vol. 87 (Springer, New York, 1994). Corrected reprint of the 1982 original.Google Scholar
Cohn, P. M., Skew fields: Theory of general division rings, Encyclopedia of Mathematics and its Applications, vol. 57 (Cambridge University Press, Cambridge, 1995).CrossRefGoogle Scholar
Degrijse, D., Amenable groups of finite cohomological dimension and the zero divisor conjecture, Preprint (2016), arXiv:1609.07635.Google Scholar
Fel'dman, G. L., The homological dimension of group algebras of solvable groups, Izv. Akad. Nauk SSSR Ser. Mat. 35 (1971), 1225–1236.Google Scholar
Gräter, J., Free division rings of fractions of crossed products of groups with Conradian left-orders, Forum Math. 32 (2020), 739–772.CrossRefGoogle Scholar
Grigorčuk, R. I., On Burnside's problem on periodic groups, Funktsional. Anal. i Prilozhen. 14 (1980), 53–54.Google Scholar
Hall, P., On the finiteness of certain soluble groups, Proc. Lond. Math. Soc. (3) 9 (1959), 595–622.CrossRefGoogle Scholar
Hillman, J. A., Elementary amenable groups and $4$-manifolds with Euler characteristic $0$, J. Aust. Math. Soc. Ser. A 50 (1991), 160–170.CrossRefGoogle Scholar
Hillman, J. A. and Linnell, P. A., Elementary amenable groups of finite Hirsch length are locally-finite by virtually-solvable, J. Aust. Math. Soc. Ser. A 52 (1992), 237–241.CrossRefGoogle Scholar
Hughes, I., Division rings of fractions for group rings, Comm. Pure Appl. Math. 23 (1970), 181–188.CrossRefGoogle Scholar
Italiano, G., Martelli, B. and Migliorini, M., Hyperbolic 5-manifolds that fiber over $S^1$, Invent. Math. 231 (2023), 1–38.CrossRefGoogle Scholar
Jaikin-Zapirain, A., The universality of Hughes-free division rings, Selecta Math. (N.S.) 27 (2021), article 74.CrossRefGoogle Scholar
Juschenko, K., Non-elementary amenable subgroups of automata groups, J. Topol. Anal. 10 (2018), 35–45.CrossRefGoogle Scholar
Kammeyer, H., $L^2$-invariants of nonuniform lattices in semisimple Lie groups, Algebr. Geom. Topol. 14 (2014), 2475–2509.CrossRefGoogle Scholar
Kielak, D., The Bieri-Neumann-Strebel invariants via Newton polytopes, Invent. Math. 219 (2020), 1009–1068.CrossRefGoogle Scholar
Kielak, D., Fibring over the circle via group homology, in Manifolds and groups, Oberwolfach Reports, vol. 17 (European Mathematical Society, 2020), 428–431.Google Scholar
Kielak, D., Residually finite rationally solvable groups and virtual fibring, J. Amer. Math. Soc. 33 (2020), 451–486.CrossRefGoogle Scholar
Koberda, T. and Suciu, A. I., Residually finite rationally $p$ groups, Commun. Contemp. Math. 22 (2020), 1950016.CrossRefGoogle Scholar
Kropholler, P. and Lorensen, K., Group-graded rings satisfying the strong rank condition, J. Algebra 539 (2019), 326–338.CrossRefGoogle Scholar
Kropholler, R., Hyperbolic groups with finitely presented subgroups not of type $F_3$, Geom. Dedicata 213 (2021), 589–619.CrossRefGoogle Scholar
Linnell, P. A., Division rings and group von Neumann algebras, Forum Math. 5 (1993), 561–576.CrossRefGoogle Scholar
Llosa Isenrich, C., Martelli, B. and Py, P., Hyperbolic groups containing subgroups of type $\mathscr {F}_{3}$ not $\mathscr {F}_{4}$, J. Differential Geom. 127 (2024), 1121–1147.CrossRefGoogle Scholar
Llosa Isenrich, C. and Py, P., Subgroups of hyperbolic groups, finiteness properties and complex hyperbolic lattices, Invent. Math. 235 (2024), 233–254.CrossRefGoogle Scholar
Lodha, Y., A hyperbolic group with a finitely presented subgroup that is not of type FP3, in Geometric and cohomological group theory, London Mathematical Society Lecture Note series, vol. 444 (Cambridge University Press, Cambridge, 2018), 67–81.Google Scholar
Lück, W., $L^2$-invariants: theory and applications to geometry and K-theory (Springer, Berlin, 2002).CrossRefGoogle Scholar
McConnell, J. C. and Robson, J. C., Noncommutative Noetherian rings, revised edition, Graduate Studies in Mathematics, vol. 30 (American Mathematical Society, Providence, RI, 2001), with the cooperation of L. W. Small.Google Scholar
Minasyan, A., Virtual retraction properties in groups, Int. Math. Res. Not. IMRN 2021 (2021), 13434–13477.CrossRefGoogle Scholar
Passman, D. S., The algebraic structure of group rings, Pure and Applied Mathematics (Wiley, 1977).Google Scholar
Rips, E., Subgroups of small cancellation groups, Bull. Lond. Math. Soc. 14 (1982), 45–47.CrossRefGoogle Scholar
Sikorav, J.-C., Homologie de Novikov associée à une classe de cohomologie de degré un, Thèse d’État, Université Paris-Sud (Orsay) (1987).Google Scholar
Stallings, J., On fibering certain 3-manifolds, in Topology of 3-manifolds and related topics (Proc. The Univ. of Georgia Institute, 1961) (Prentice-Hall, Englewood Cliffs, NJ, 1962), 95–100.Google Scholar