Hostname: page-component-669899f699-2mbcq Total loading time: 0 Render date: 2025-04-28T02:43:05.940Z Has data issue: false hasContentIssue false
  • English
  • Français

Generalized D-symmetric Operators II

Published online by Cambridge University Press:  20 November 2018

S. Bouali  and
M. Ech-chad
S. Bouali
Affiliation:
Department of Mathematics and Informatics, Faculty of Sciences Kénitra, B. P. 133 Kénitra, Moroccoe-mail: [email protected]
M. Ech-chad
Affiliation:
Lycée mixte de Missour, 33250 Missour, Moroccoe-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $H$ be a separable, infinite-dimensional, complex Hilbert space and let $A,\,B\,\in \,\mathcal{L}\left( H \right)$, where 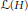$\mathcal{L}(H)$ is the algebra of all bounded linear operators on $H$. Let 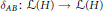${{\delta }_{AB}}\,:\mathcal{L}\left( H \right)\to \mathcal{L}\left( H \right)$ denote the generalized derivation 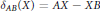${{\delta }_{AB}}\left( X \right)\,=\,AX\,-\,XB$. This note will initiate a study on the class of pairs 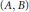$\left( A,\,B \right)$ such that 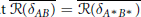$\overline{R\left( {{\delta }_{AB}} \right)}\,=\,\overline{R\left( {{\delta }_{A*\,B*}} \right)}$.

Keywords

47B4747B1047A30generalized derivation, adjointD-symmetric operatornormal operator
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 54 , Issue 1 , 01 March 2011 , pp. 21 - 27
Copyright
Copyright © Canadian Mathematical Society 2011

References

[1] Anderson, J., Bunce, J. W., Deddens, J. A., and Williams, J. P., C*-algebras and derivation ranges. Acta Sci. Math. (Szeged) 40(1978), no. 3–4, 211–227.Google Scholar
[2] Anderson, J. and Foias, C., Properties which normal operators share with normal derivation and related operators. Pacific J. Math. 61(1975), no. 2, 313–325.Google Scholar
[3] Benlarbi, M., Bouali, S., and Cherki, S., Une remarque sur l’orthogonalité de l’image au noyau d’une dérivation généralisée. Proc. Amer. Math. Soc. 126(1998), no. 1, 167–171. doi:10.1090/S0002-9939-98-03996-3Google Scholar
[4] Bouali, S. and Charles, J., Extension de la notion d’opérateur D-symétrique. I. Acta Sci. Math. (Szeged) 58(1993), no. 1–4, 517–525.Google Scholar
[5] Bouali, S. and Charles, J., Extension de la notion d’opérateur D-symétrique. II. Linear Algebra Appl. 225(1995), 175–185. doi:10.1016/0024-3795(94)00003-VGoogle Scholar
[6] Herrero, D. A., Approximation of Hilbert space operators. Vol. I, Research Notes in Mathematics, 72, Pitman (Advanced Publishing Program), Boston, MA, 1982.Google Scholar
[7] Rosenblum, M., On the operator equation BX – XA = Q. Duke Math. J. 23(1956), 263–269. doi:10.1215/S0012-7094-56-02324-9Google Scholar
[8] Stampfli, J. G., On self-adjoint derivation ranges. Pacific J. Math. 82(1979), no. 1, 257–277.Google Scholar
[9] Williams, J. P., Derivation ranges: open problems. In: Topics in modern operator theory, Operator Theory: Adv. Appl., 2, Birkhäuser, Basel-Boston, MA, 1981, pp. 319–328.Google Scholar
[10] Williams, J. P., On the range of a derivation. Pacific J. Math. 38(1971), 273–279.Google Scholar