Hostname: page-component-669899f699-qzcqf Total loading time: 0 Render date: 2025-04-29T01:38:23.207Z Has data issue: false hasContentIssue false
  • English
  • Français

THE SHARP BOUND OF THE SECOND HANKEL DETERMINANT OF LOGARITHMIC COEFFICIENTS FOR STARLIKE AND CONVEX FUNCTIONS

Part of: Geometric function theory

Published online by Cambridge University Press:  06 November 2024

VASUDEVARAO ALLU Open the ORCID record for VASUDEVARAO ALLU [Opens in a new window]  and
AMAL SHAJI
VASUDEVARAO ALLU*
Affiliation:
Department of Mathematics, School of Basic Sciences, Indian Institute of Technology Bhubaneswar, Bhubaneswar 752050, Odisha, India
AMAL SHAJI
Affiliation:
Department of Mathematics, School of Basic Sciences, Indian Institute of Technology Bhubaneswar, Bhubaneswar 752050, Odisha, India e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 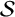$\mathcal {S}$ denote the class of univalent functions in the open unit disc 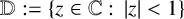$\mathbb {D}:=\{z\in \mathbb {C}:\, |z|<1\}$ with the form 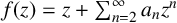$f(z)= z+\sum _{n=2}^{\infty }a_n z^n$. The logarithmic coefficients $\gamma _{n}$ of 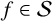$f\in \mathcal {S}$ are defined by 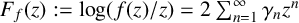$F_{f}(z):= \log (f(z)/z)=2\sum _{n=1}^{\infty }\gamma _{n}z^{n}$. The second Hankel determinant for logarithmic coefficients is defined by

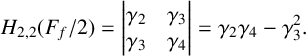$$ \begin{align*} H_{2,2}(F_f/2) = \begin{vmatrix} \gamma_2 & \gamma_3 \\ \gamma_3 & \gamma_4 \end{vmatrix} =\gamma_2\gamma_4 -\gamma_3^2. \end{align*} $$

We obtain sharp upper bounds of the second Hankel determinant of logarithmic coefficients for starlike and convex functions.

Keywords

univalent functionslogarithmic coefficientsHankel determinantstarlike and convex functionsSchwarz function

MSC classification

Primary: 30C45: Special classes of univalent and multivalent functions (starlike, convex, bounded rotation, etc.)
Secondary: 30C50: Coefficient problems for univalent and multivalent functions 30C55: General theory of univalent and multivalent functions
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , First View , pp. 1 - 9
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

The research of the second author is supported by UGC-JRF.

References

Ali, M. F. and Allu, V., ‘On logarithmic coefficients of some close-to-convex functions’, Proc. Amer. Math. Soc. 146 (2017), 1131–1142.CrossRefGoogle Scholar
Ali, M. F. and Allu, V., ‘Logarithmic coefficients of some close-to-convex functions’, Bull. Aust. Math. Soc. 95 (2017), 228–237.CrossRefGoogle Scholar
Allu, V. and Arora, V., ‘Second Hankel determinant of logarithmic coefficients of certain analytic functions’, Rocky Mountain J. Math. 54(2) (2024), 343–359.CrossRefGoogle Scholar
Allu, V., Arora, V. and Shaji, A., ‘On the second Hankel determinant of logarithmic coefficients for certain univalent functions’, Mediterr. J. Math.. 20 (2023), Article no. 81.CrossRefGoogle Scholar
Allu, V., Lecko, A. and Thomas, D. K., ‘Hankel, Toeplitz and Hermitian–Toeplitz determinants for Ozaki close-to-convex functions’, Mediterr. J. Math. 19 (2022), Article no. 22.CrossRefGoogle Scholar
Allu, V. and Shaji, A., ‘Second Hankel determinant of logarithmic inverse coefficients of convex and starlike functions’, Bull. Aust. Math. Soc. (2024), 1–12.CrossRefGoogle Scholar
Carlson, F., ‘Sur les coefficients d’une fonction bornée dans le cercle unité’, Ark. Mat. Astr. Fys. 27A (1940), 1–8.Google Scholar
Cho, N. E., Kowalczyk, B., Kwon, O., Lecko, A. and Sim, Y., ‘On the third logarithmic coefficient in some subclasses of close-to-convex functions’, Rev. R. Acad. Cienc. Exactas Fís. Nat. (Esp.) 114 (2020), Article no. 52.Google Scholar
de Branges, L., ‘A proof of the Bieberbach conjecture’, Acta Math. 154 (1985), 137–152.CrossRefGoogle Scholar
Duren, P. L., Univalent Functions, Grundlehren der mathematischen Wissenschaften, 259 (Springer, New York–Berlin–Heidelberg–Tokyo, 1983).Google Scholar
Efraimidis, I., ‘A generalization of Livingston’s coefficient inequalities for functions with positive real part’, J. Math. Anal. Appl. 435(1) (2016), 369–379.CrossRefGoogle Scholar
Eker, S. S., Lecko, A., Çekiç, B. and Şeker, B., ‘The second Hankel determinant of logarithmic coefficients for strongly Ozaki close-to-convex functions’, Bull. Malays. Math. Sci. Soc. 46 (2023), Article no. 183.Google Scholar
Kowalczyk, B. and Lecko, A., ‘Second Hankel determinant of logarithmic coefficients of convex and starlike functions’, Bull. Aust. Math. Soc. 105 (2022), 458–467.CrossRefGoogle Scholar
Kowalczyk, B. and Lecko, A., ‘Second Hankel determinant of logarithmic coefficients of convex and starlike functions of order alpha’, Bull. Malays. Math. Sci. Soc. 45 (2022), 727–740.CrossRefGoogle Scholar
Kowalczyk, B. and Lecko, A., ‘The second Hankel determinant of the logarithmic coefficients of strongly starlike and strongly convex functions’, Rev. R. Acad. Cienc. Exactas Fís. Nat. Ser. A Mat. RACSAM 117(91) (2023), Article no. 91.Google Scholar
Livingston, A. E., ‘The coefficients of multivalent close-to-convex functions’, Proc. Amer. Math. Soc. 21 (1969), 545–552.CrossRefGoogle Scholar
Milin, I. M., Univalent Functions and Orthonormal Systems, Translations of Mathematical Monographs, 49 (American Mathematical Society, Providence, RI, 1977).Google Scholar
Pommerenke, C., ‘On the coefficients and Hankel determinants of univalent functions’, J. Lond. Math. Soc. (2) 41 (1966), 111–122.CrossRefGoogle Scholar
Pommerenke, C., ‘On the Hankel determinants of univalent functions’, Mathematika 14 (1967), 108–112.CrossRefGoogle Scholar
Pranav Kumar, U. and Vasudevarao, A., ‘Logarithmic coefficients for certain subclasses of close-to-convex functions’, Monatsh. Math. 187 (2018), 543–563.CrossRefGoogle Scholar
Thomas, D. K., ‘On the logarithmic coefficients of close-to-convex functions’, Proc. Amer. Math. Soc. 144 (2016), 1681–1687.CrossRefGoogle Scholar
Zaprawa, P., ‘On a coefficient inequality for Carathéodory functions’, Results Math. 79 (2024), Article no. 30.CrossRefGoogle Scholar