No CrossRef data available.
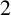 $2$ CURVE
$2$ CURVEPublished online by Cambridge University Press: 04 October 2024
Cardona and Lario [‘Twists of the genus 2 curve 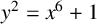 $y^2 = x^6+1$’, J. Number Theory 209 (2020), 195–211] gave a complete classification of the twists of the curve
$y^2 = x^6+1$’, J. Number Theory 209 (2020), 195–211] gave a complete classification of the twists of the curve 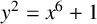 $y^2 = x^6+1$. In this paper, we study the twists of the curve whose automorphism group is defined over a biquadratic extension of the rationals. If the twists are of type B or C in the Cardona–Lario classification, we find a pair of elliptic curves whose product is isogenous with the Jacobian of the twist.
$y^2 = x^6+1$. In this paper, we study the twists of the curve whose automorphism group is defined over a biquadratic extension of the rationals. If the twists are of type B or C in the Cardona–Lario classification, we find a pair of elliptic curves whose product is isogenous with the Jacobian of the twist.
K. Jeong was supported by the National Research Foundation of Korea (NRF) grant funded by the Korea government (MSIT) (RS-2024-00341372). Y.-W. Kwon was supported by Basic Science Research Program through the National Research Foundation of Korea (NRF) funded by the Ministry of Education (2022R1I1A1A01067581). J. Park was supported by Samsung Science and Technology Foundation under Project Number SSTF-BA2001-02 and the National Research Foundation of Korea (NRF) grant funded by the Korea government (MSIT) (RS-2024-00449679).