No CrossRef data available.
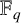 $\mathbb {F}_q$, WHERE
$\mathbb {F}_q$, WHERE 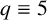 $q\equiv 5$ (mod 8)
$q\equiv 5$ (mod 8)Published online by Cambridge University Press: 27 February 2025
Arithmetic-geometric mean sequences were already studied over the real and complex numbers, and recently, Griffin et al. [‘AGM and jellyfish swarms of elliptic curves’, Amer. Math. Monthly 130(4) (2023), 355–369] considered them over finite fields 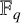 $\mathbb {F}_q$ for
$\mathbb {F}_q$ for 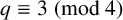 $q \equiv 3 \pmod 4$. We extend the definition of arithmetic-geometric mean sequences over
$q \equiv 3 \pmod 4$. We extend the definition of arithmetic-geometric mean sequences over 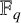 $\mathbb {F}_q$ to
$\mathbb {F}_q$ to  $q \equiv 5 \pmod 8$. We explain the connection of these sequences with graphs and explore the properties of the graphs in the case where
$q \equiv 5 \pmod 8$. We explain the connection of these sequences with graphs and explore the properties of the graphs in the case where 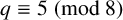 $q \equiv 5 \pmod 8$.
$q \equiv 5 \pmod 8$.
The second author was supported by the Czech Science Foundation GAČR, grant 21-00420M and a Junior Fund grant for postdoctoral positions at Charles University.