For any real polynomial 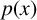$p(x)$ of even degree k, Shapiro [‘Problems around polynomials: the good, the bad and the ugly$\ldots $’, Arnold Math. J. 1(1) (2015), 91–99] proposed the conjecture that the sum of the number of real zeros of the two polynomials 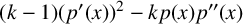$(k-1)(p{'}(x))^{2}-kp(x)p{"}(x)$ and 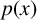$p(x)$ is larger than 0. We prove that the conjecture is true except in one case: when the polynomial $p(x)$ has no real zeros, the derivative polynomial $p{'}(x)$ has one real simple zero, that is, 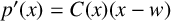$p{'}(x)=C(x)(x-w)$, where 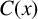$C(x)$ is a polynomial with 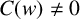$C(w)\ne 0$, and the polynomial 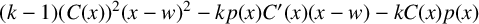$(k-1)(C(x))^2(x-w)^{2}-kp(x)C{'}(x)(x-w)-kC(x)p(x)$ has no real zeros.